Question and Answers Forum

All Questions      Topic List

Integration Questions

Previous in All Question      Next in All Question      

Previous in Integration      Next in Integration      

Question Number 106932 by Ar Brandon last updated on 07/Aug/20

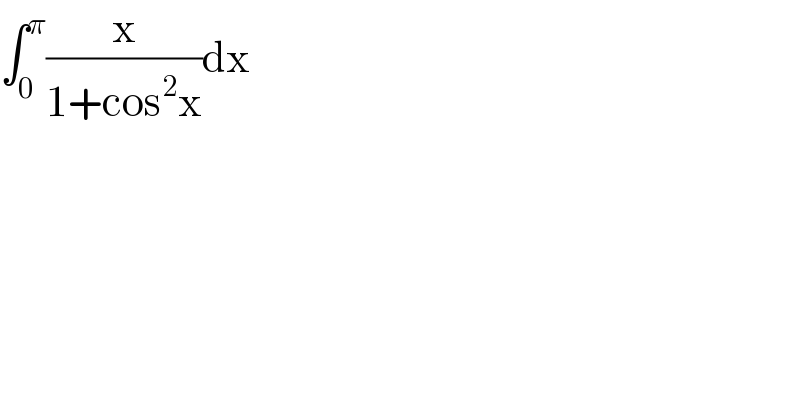
∫_0 ^π (x/(1+cos^2 x))dx

$$\int_{\mathrm{0}} ^{\pi} \frac{\mathrm{x}}{\mathrm{1}+\mathrm{cos}^{\mathrm{2}} \mathrm{x}}\mathrm{dx} \\ $$

Answered by mathmax by abdo last updated on 08/Aug/20

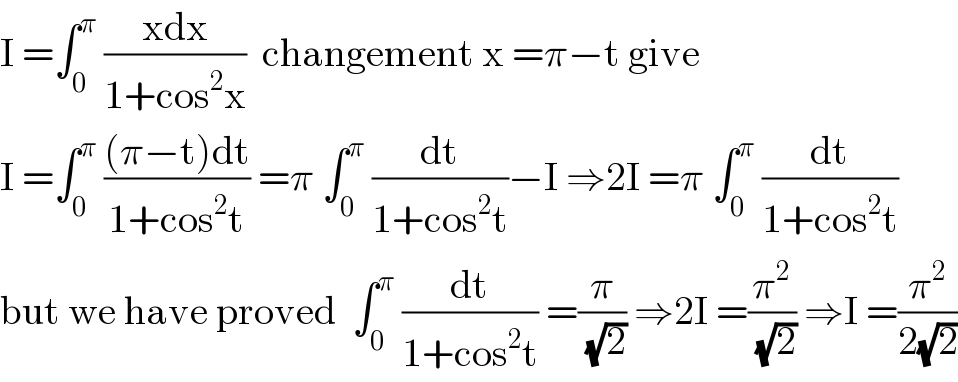
I =∫_0 ^π ((xdx)/(1+cos^2 x)) changement x =π−t give I =∫_0 ^π (((π−t)dt)/(1+cos^2 t)) =π ∫_0 ^π (dt/(1+cos^2 t))−I ⇒2I =π ∫_0 ^π (dt/(1+cos^2 t)) but we have proved ∫_0 ^π (dt/(1+cos^2 t)) =(π/(√2)) ⇒2I =(π^2 /(√2)) ⇒I =(π^2 /(2(√2)))

$$\mathrm{I}\:=\int_{\mathrm{0}} ^{\pi} \:\frac{\mathrm{xdx}}{\mathrm{1}+\mathrm{cos}^{\mathrm{2}} \mathrm{x}}\:\:\mathrm{changement}\:\mathrm{x}\:=\pi−\mathrm{t}\:\mathrm{give} \\ $$$$\mathrm{I}\:=\int_{\mathrm{0}} ^{\pi} \:\frac{\left(\pi−\mathrm{t}\right)\mathrm{dt}}{\mathrm{1}+\mathrm{cos}^{\mathrm{2}} \mathrm{t}}\:=\pi\:\int_{\mathrm{0}} ^{\pi} \:\frac{\mathrm{dt}}{\mathrm{1}+\mathrm{cos}^{\mathrm{2}} \mathrm{t}}−\mathrm{I}\:\Rightarrow\mathrm{2I}\:=\pi\:\int_{\mathrm{0}} ^{\pi} \:\frac{\mathrm{dt}}{\mathrm{1}+\mathrm{cos}^{\mathrm{2}} \mathrm{t}} \\ $$$$\mathrm{but}\:\mathrm{we}\:\mathrm{have}\:\mathrm{proved}\:\:\int_{\mathrm{0}} ^{\pi} \:\frac{\mathrm{dt}}{\mathrm{1}+\mathrm{cos}^{\mathrm{2}} \mathrm{t}}\:=\frac{\pi}{\sqrt{\mathrm{2}}}\:\Rightarrow\mathrm{2I}\:=\frac{\pi^{\mathrm{2}} }{\sqrt{\mathrm{2}}}\:\Rightarrow\mathrm{I}\:=\frac{\pi^{\mathrm{2}} }{\mathrm{2}\sqrt{\mathrm{2}}} \\ $$

Answered by Dwaipayan Shikari last updated on 08/Aug/20

∫_0 ^π (x/(1+cos^2 x))=∫((π−x)/(1+cos^2 x))=I 2I=∫_0 ^π (π/(1+cos^2 x)) 2I=π∫_0 ^π ((sec^2 x)/(sec^2 x+1)) 2I=π∫_0 ^π (dt/(t^2 +2)) (tanx=t 2I=(π/(√2))[tan^(−1) (((tanx)/(√2)))]_0 ^π =(π/(√2)).π=(π^2 /(√2)) I=(π^2 /(2(√2)))

$$\int_{\mathrm{0}} ^{\pi} \frac{{x}}{\mathrm{1}+{cos}^{\mathrm{2}} {x}}=\int\frac{\pi−{x}}{\mathrm{1}+{cos}^{\mathrm{2}} {x}}={I} \\ $$$$\mathrm{2}{I}=\int_{\mathrm{0}} ^{\pi} \frac{\pi}{\mathrm{1}+{cos}^{\mathrm{2}} {x}} \\ $$$$\mathrm{2}{I}=\pi\int_{\mathrm{0}} ^{\pi} \frac{{sec}^{\mathrm{2}} {x}}{{sec}^{\mathrm{2}} {x}+\mathrm{1}} \\ $$$$\mathrm{2}{I}=\pi\int_{\mathrm{0}} ^{\pi} \frac{{dt}}{{t}^{\mathrm{2}} +\mathrm{2}}\:\:\:\:\:\:\:\:\:\:\:\:\:\left({tanx}={t}\right. \\ $$$$\mathrm{2}{I}=\frac{\pi}{\sqrt{\mathrm{2}}}\left[{tan}^{−\mathrm{1}} \left(\frac{\mathrm{tanx}}{\sqrt{\mathrm{2}}}\right)\right]_{\mathrm{0}} ^{\pi} =\frac{\pi}{\sqrt{\mathrm{2}}}.\pi=\frac{\pi^{\mathrm{2}} }{\sqrt{\mathrm{2}}} \\ $$$${I}=\frac{\pi^{\mathrm{2}} }{\mathrm{2}\sqrt{\mathrm{2}}} \\ $$

Commented by Ar Brandon last updated on 08/Aug/20

������

Answered by Ar Brandon last updated on 08/Aug/20

J=∫_0 ^π (x/(1+cos^2 x))dx =∫_0 ^π ((π−x)/(1+cos^2 x))dx=∫_0 ^π (π/(1+cos^2 x))dx−J 2J=∫_0 ^π (π/(1+cos^2 x))dx=π∫_0 ^π ((sec^2 x)/(sec^2 x+1))dx =π∫_0 ^π ((sec^2 x)/(tan^2 x+2))dx=π∫_0 ^π ((d(tanx))/(tan^2 x+2))dx =π[(1/(√2))Arctan(((tanx)/(√2)))]_0 ^π =(π/(√2))[Arctan(−(0/(√2)))−Arctan((0/(√2)) )]=((π(π−0))/(√2))=(π^2 /(√2)) J=(π^2 /(2(√2)))

$$\mathcal{J}=\int_{\mathrm{0}} ^{\pi} \frac{\mathrm{x}}{\mathrm{1}+\mathrm{cos}^{\mathrm{2}} \mathrm{x}}\mathrm{dx} \\ $$$$\:\:\:\:=\int_{\mathrm{0}} ^{\pi} \frac{\pi−\mathrm{x}}{\mathrm{1}+\mathrm{cos}^{\mathrm{2}} \mathrm{x}}\mathrm{dx}=\int_{\mathrm{0}} ^{\pi} \frac{\pi}{\mathrm{1}+\mathrm{cos}^{\mathrm{2}} \mathrm{x}}\mathrm{dx}−\mathcal{J} \\ $$$$\mathrm{2}\mathcal{J}=\int_{\mathrm{0}} ^{\pi} \frac{\pi}{\mathrm{1}+\mathrm{cos}^{\mathrm{2}} \mathrm{x}}\mathrm{dx}=\pi\int_{\mathrm{0}} ^{\pi} \frac{\mathrm{sec}^{\mathrm{2}} \mathrm{x}}{\mathrm{sec}^{\mathrm{2}} \mathrm{x}+\mathrm{1}}\mathrm{dx} \\ $$$$\:\:\:\:\:\:\:=\pi\int_{\mathrm{0}} ^{\pi} \frac{\mathrm{sec}^{\mathrm{2}} \mathrm{x}}{\mathrm{tan}^{\mathrm{2}} \mathrm{x}+\mathrm{2}}\mathrm{dx}=\pi\int_{\mathrm{0}} ^{\pi} \frac{\mathrm{d}\left(\mathrm{tanx}\right)}{\mathrm{tan}^{\mathrm{2}} \mathrm{x}+\mathrm{2}}\mathrm{dx} \\ $$$$\:\:\:\:\:\:\:=\pi\left[\frac{\mathrm{1}}{\sqrt{\mathrm{2}}}\mathrm{Arctan}\left(\frac{\mathrm{tanx}}{\sqrt{\mathrm{2}}}\right)\right]_{\mathrm{0}} ^{\pi} \\ $$$$\:\:\:\:\:\:\:=\frac{\pi}{\sqrt{\mathrm{2}}}\left[\mathrm{Arctan}\left(−\frac{\mathrm{0}}{\sqrt{\mathrm{2}}}\right)−\mathrm{Arctan}\left(\frac{\mathrm{0}}{\sqrt{\mathrm{2}}}\:\right)\right]=\frac{\pi\left(\pi−\mathrm{0}\right)}{\sqrt{\mathrm{2}}}=\frac{\pi^{\mathrm{2}} }{\sqrt{\mathrm{2}}} \\ $$$$\mathcal{J}=\frac{\pi^{\mathrm{2}} }{\mathrm{2}\sqrt{\mathrm{2}}} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com