
Question Number 211150 by Spillover last updated on 29/Aug/24
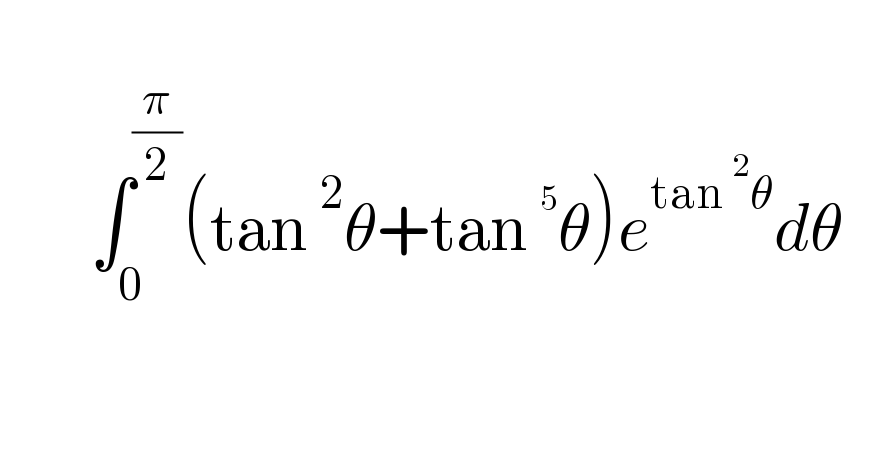
$$ \\ $$$$\:\:\:\:\:\:\:\:\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \left(\mathrm{tan}\:^{\mathrm{2}} \theta+\mathrm{tan}\:^{\mathrm{5}} \theta\right){e}^{\mathrm{tan}\:^{\mathrm{2}} \theta} {d}\theta \\ $$$$ \\ $$$$ \\ $$
Commented by Ghisom last updated on 30/Aug/24
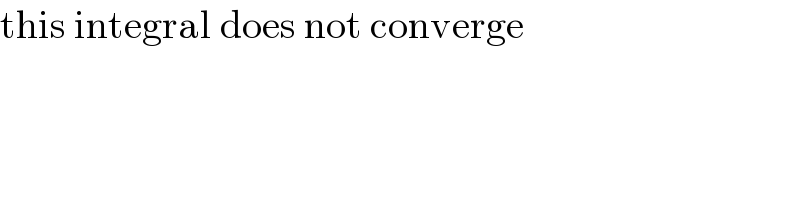
$$\mathrm{this}\:\mathrm{integral}\:\mathrm{does}\:\mathrm{not}\:\mathrm{converge} \\ $$
Answered by MathematicalUser2357 last updated on 11/Sep/24
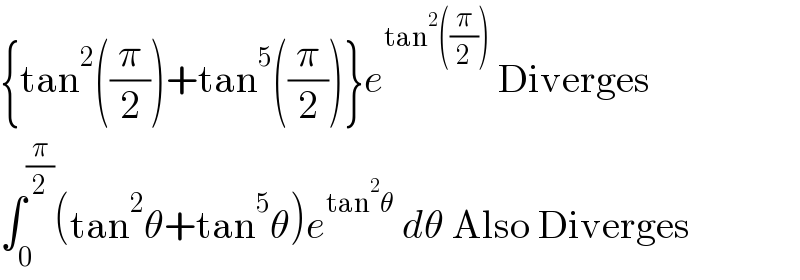
$$\left\{\mathrm{tan}^{\mathrm{2}} \left(\frac{\pi}{\mathrm{2}}\right)+\mathrm{tan}^{\mathrm{5}} \left(\frac{\pi}{\mathrm{2}}\right)\right\}{e}^{\mathrm{tan}^{\mathrm{2}} \left(\frac{\pi}{\mathrm{2}}\right)} \:\mathrm{Diverges} \\ $$$$\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \left(\mathrm{tan}^{\mathrm{2}} \theta+\mathrm{tan}^{\mathrm{5}} \theta\right){e}^{\mathrm{tan}^{\mathrm{2}} \theta} \:{d}\theta\:\mathrm{Also}\:\mathrm{Diverges} \\ $$
