Question and Answers Forum

All Questions      Topic List

Integration Questions

Previous in All Question      Next in All Question      

Previous in Integration      Next in Integration      

Question Number 97109 by student work last updated on 06/Jun/20

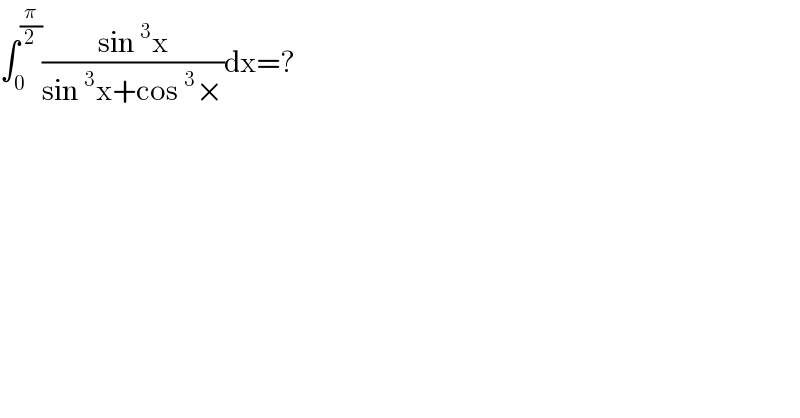
∫_0 ^(π/2) ((sin^3 x)/(sin^3 x+cos^3 ×))dx=?

$$\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \frac{\mathrm{sin}\:^{\mathrm{3}} \mathrm{x}}{\mathrm{sin}\:^{\mathrm{3}} \mathrm{x}+\mathrm{cos}\:^{\mathrm{3}} ×}\mathrm{dx}=? \\ $$

Answered by Sourav mridha last updated on 06/Jun/20

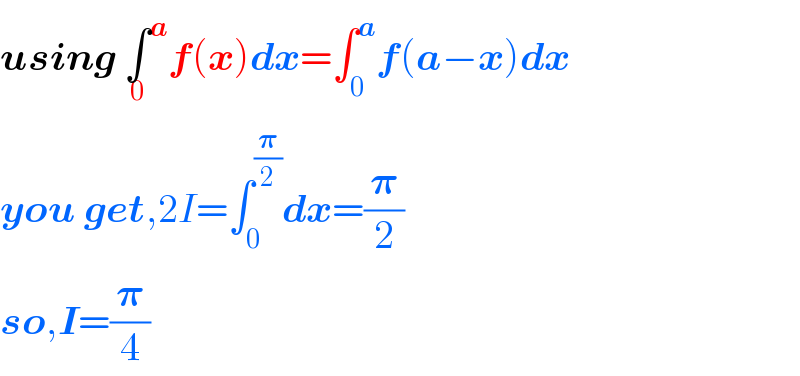
using ∫^a _0 f(x)dx=∫_0 ^a f(a−x)dx you get,2I=∫_0 ^(𝛑/2) dx=(𝛑/2) so,I=(𝛑/4)

$$\boldsymbol{{using}}\:\underset{\mathrm{0}} {\int}^{\boldsymbol{{a}}} \boldsymbol{{f}}\left(\boldsymbol{{x}}\right)\boldsymbol{{dx}}=\int_{\mathrm{0}} ^{\boldsymbol{{a}}} \boldsymbol{{f}}\left(\boldsymbol{{a}}−\boldsymbol{{x}}\right)\boldsymbol{{dx}} \\ $$$$\boldsymbol{{you}}\:\boldsymbol{{get}},\mathrm{2}{I}=\int_{\mathrm{0}} ^{\frac{\boldsymbol{\pi}}{\mathrm{2}}} \boldsymbol{{dx}}=\frac{\boldsymbol{\pi}}{\mathrm{2}} \\ $$$$\boldsymbol{{so}},\boldsymbol{{I}}=\frac{\boldsymbol{\pi}}{\mathrm{4}} \\ $$

Commented by student work last updated on 06/Jun/20

how is the solution?

$$\mathrm{how}\:\mathrm{is}\:\mathrm{the}\:\mathrm{solution}? \\ $$

Answered by Sourav mridha last updated on 06/Jun/20

I=∫_0 ^(π/2) ((sin^3 (x))/(sin^3 (x)+cos^3 (x)))dx..(i) also I=∫_0 ^(π/2) ((sin^3 ((π/2)−x))/(sin^3 ((π/2)−x)+cos^3 ((π/2)−x)))dx =∫_0 ^(π/2) ((cos^3 (x))/(cos^3 (x)+sin^3 (x)))dx..(ii) now (i)+(ii),2I=∫_0 ^(π/2) dx=[(π/2)−0] so I=(π/4)

$$\boldsymbol{{I}}=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \frac{\mathrm{sin}^{\mathrm{3}} \left(\mathrm{x}\right)}{\mathrm{sin}^{\mathrm{3}} \left(\mathrm{x}\right)+\mathrm{cos}^{\mathrm{3}} \left(\mathrm{x}\right)}\mathrm{dx}..\left(\mathrm{i}\right) \\ $$$$\mathrm{also}\:\boldsymbol{{I}}=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \frac{\mathrm{sin}^{\mathrm{3}} \left(\frac{\pi}{\mathrm{2}}−\mathrm{x}\right)}{\mathrm{sin}^{\mathrm{3}} \left(\frac{\pi}{\mathrm{2}}−\mathrm{x}\right)+\mathrm{cos}^{\mathrm{3}} \left(\frac{\pi}{\mathrm{2}}−\mathrm{x}\right)}\mathrm{dx} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \frac{\mathrm{cos}^{\mathrm{3}} \left(\mathrm{x}\right)}{\mathrm{cos}^{\mathrm{3}} \left(\mathrm{x}\right)+\mathrm{sin}^{\mathrm{3}} \left(\mathrm{x}\right)}\mathrm{dx}..\left(\mathrm{ii}\right) \\ $$$$\mathrm{now}\:\left(\mathrm{i}\right)+\left(\mathrm{ii}\right),\mathrm{2}\boldsymbol{{I}}=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \mathrm{dx}=\left[\frac{\pi}{\mathrm{2}}−\mathrm{0}\right] \\ $$$$\:\:\:\:\mathrm{so}\:\boldsymbol{{I}}=\frac{\pi}{\mathrm{4}}\:\:\:\:\:\: \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com