
Question Number 142724 by lapache last updated on 04/Jun/21
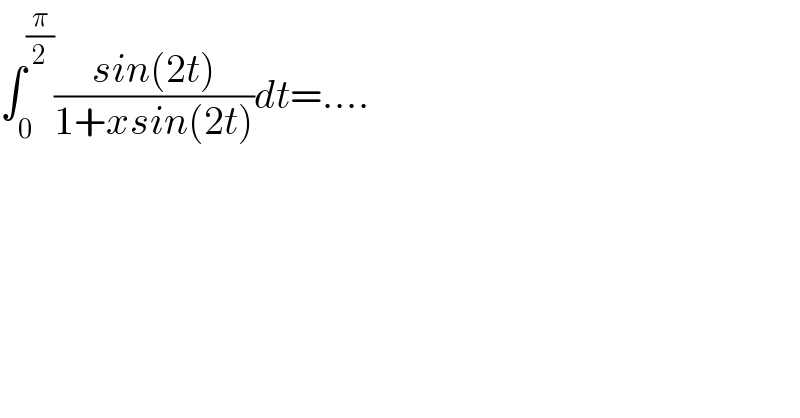
$$\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \frac{{sin}\left(\mathrm{2}{t}\right)}{\mathrm{1}+{xsin}\left(\mathrm{2}{t}\right)}{dt}=.... \\ $$
Answered by Ar Brandon last updated on 04/Jun/21
![I=∫_0 ^(π/2) ((sin2t)/(1+xsin2t))dt=(1/x)∫_0 ^(π/2) ((xsin2t)/(1+xsin2t))dt =(1/x)∫_0 ^(π/2) (1−(1/(1+xsin2t)))dt=(π/(2x))−(1/x)∫_0 ^(π/2) (dt/(1+xsin2t)) =(π/(2x))−(1/x)∫_0 ^(π/2) ((sec^2 t)/(sec^2 t+xtant))dt=(π/(2x))−(1/x)∫_0 ^∞ (dj/(j^2 +xj+1)) =(π/(2x))−(1/x)∫_0 ^∞ (dj/((j+(x/2))^2 +1−(x^2 /4)))=(π/(2x))−(2/(x(√(4−x^2 ))))[arctan(((2j+x)/( (√(4−x^2 )))))]_0 ^∞ =(π/(2x))−(2/(x(√(4−x^2 ))))((π/2)−arctan((x/( (√(4−x^2 ))))))](Q142727.png)
$$\mathrm{I}=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \frac{\mathrm{sin2t}}{\mathrm{1}+\mathrm{xsin2t}}\mathrm{dt}=\frac{\mathrm{1}}{\mathrm{x}}\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \frac{\mathrm{xsin2t}}{\mathrm{1}+\mathrm{xsin2t}}\mathrm{dt} \\ $$$$\:\:=\frac{\mathrm{1}}{\mathrm{x}}\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \left(\mathrm{1}−\frac{\mathrm{1}}{\mathrm{1}+\mathrm{xsin2t}}\right)\mathrm{dt}=\frac{\pi}{\mathrm{2x}}−\frac{\mathrm{1}}{\mathrm{x}}\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \frac{\mathrm{dt}}{\mathrm{1}+\mathrm{xsin2t}} \\ $$$$\:\:=\frac{\pi}{\mathrm{2x}}−\frac{\mathrm{1}}{\mathrm{x}}\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \frac{\mathrm{sec}^{\mathrm{2}} \mathrm{t}}{\mathrm{sec}^{\mathrm{2}} \mathrm{t}+\mathrm{xtant}}\mathrm{dt}=\frac{\pi}{\mathrm{2x}}−\frac{\mathrm{1}}{\mathrm{x}}\int_{\mathrm{0}} ^{\infty} \frac{\mathrm{d}{j}}{{j}^{\mathrm{2}} +\mathrm{x}{j}+\mathrm{1}} \\ $$$$\:\:=\frac{\pi}{\mathrm{2x}}−\frac{\mathrm{1}}{\mathrm{x}}\int_{\mathrm{0}} ^{\infty} \frac{\mathrm{d}{j}}{\left({j}+\frac{\mathrm{x}}{\mathrm{2}}\right)^{\mathrm{2}} +\mathrm{1}−\frac{\mathrm{x}^{\mathrm{2}} }{\mathrm{4}}}=\frac{\pi}{\mathrm{2x}}−\frac{\mathrm{2}}{\mathrm{x}\sqrt{\mathrm{4}−\mathrm{x}^{\mathrm{2}} }}\left[\mathrm{arctan}\left(\frac{\mathrm{2}{j}+\mathrm{x}}{\:\sqrt{\mathrm{4}−\mathrm{x}^{\mathrm{2}} }}\right)\right]_{\mathrm{0}} ^{\infty} \\ $$$$\:\:=\frac{\pi}{\mathrm{2x}}−\frac{\mathrm{2}}{\mathrm{x}\sqrt{\mathrm{4}−\mathrm{x}^{\mathrm{2}} }}\left(\frac{\pi}{\mathrm{2}}−\mathrm{arctan}\left(\frac{\mathrm{x}}{\:\sqrt{\mathrm{4}−\mathrm{x}^{\mathrm{2}} }}\right)\right) \\ $$
Answered by mathmax by abdo last updated on 06/Jun/21
![I(x)=∫_0 ^(π/2) ((sin(2t))/(1+xsin(2t)))dt ⇒I(x)=_(2t=u) (1/2) ∫_0 ^π ((sinu)/(1+xsinu))du =(1/(2x))∫_0 ^π ((xsinu+1−1)/(1+xsinu))du =(π/(2x))−(1/(2x))∫_0 ^π (du/(1+xsinu)) changement tan((u/2))=y give ∫_0 ^π (du/(1+xsinu)) =∫_0 ^∞ ((2dy)/((1+y^2 )(1+x((2y)/(1+y^2 ))))) H=∫_0 ^∞ ((2dy)/(1+y^2 +2xy)) =∫_0 ^∞ ((2dy)/(y^2 +2xy +1)) =∫_0 ^∞ ((2dy)/((y+x)^2 +1−x^2 )) case 1 1−x^2 >0 ⇒∣x∣<1 ⇒H=_(y+x=(√(1−x^2 ))z) ∫_(x/( (√(1−x^2 )))) ^∞ ((2(√(1−x^2 ))dz)/((1−x^2 )(1+z^2 ))) =(2/( (√(1−x^2 ))))[arctanz]_(x/( (√(1−x^2 )))) ^∞ =(2/( (√(1−x^2 ))))((π/2)−arctan((x/( (√(1−x^2 )))))) =(π/( (√(1−x^2 ))))−(2/( (√(1−x^2 ))))arctan((x/( (√(1−x^2 ))))) ⇒ I(x)=(π/(2x))−(π/(2x(√(1−x^2 ))))−(1/(x(√(1−x^2 ))))arctan((x/( (√(1−x^2 ))))) case 2 1−x^2 <0 ⇒H=∫_0 ^∞ ((2dy)/((y+x)^2 −(x^2 −1))) =_(y+x=(√(x^2 −1))u) ∫_(x/( (√(x^2 −1)))) ^∞ ((2(√(x^2 −1))du)/((x^2 −1)(u^2 −1))) =(2/( (√(x^2 −1))))∫_(x/( (√(x^2 −1)))) ^∞ (du/((u−1)(u+1))) =(1/( (√(x^2 −1))))∫_(x/( (√(x^2 −1)))) ^∞ ((1/(u−1))−(1/(u+1))) =(1/( (√(x^2 −1))))[log∣((u−1)/(u+1))∣]_(x/( (√(x^2 −1)))) ^∞ =−(1/( (√(x^2 −1))))log∣(((x/( (√(x^2 −1))))−1)/((x/( (√(x^2 −1))))+1))∣ =−(1/( (√(x^2 −1))))log∣((x−(√(x^2 −1)))/(x+(√(x^2 −1))))∣ ⇒ I(x)=(π/(2x))+(1/(2x(√(x^2 −1))))log∣((x−(√(x^2 −1)))/(x+(√(x^2 −1))))∣](Q142839.png)
$$\mathrm{I}\left(\mathrm{x}\right)=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \:\frac{\mathrm{sin}\left(\mathrm{2t}\right)}{\mathrm{1}+\mathrm{xsin}\left(\mathrm{2t}\right)}\mathrm{dt}\:\Rightarrow\mathrm{I}\left(\mathrm{x}\right)=_{\mathrm{2t}=\mathrm{u}} \frac{\mathrm{1}}{\mathrm{2}}\:\:\int_{\mathrm{0}} ^{\pi} \:\frac{\mathrm{sinu}}{\mathrm{1}+\mathrm{xsinu}}\mathrm{du} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2x}}\int_{\mathrm{0}} ^{\pi} \:\frac{\mathrm{xsinu}+\mathrm{1}−\mathrm{1}}{\mathrm{1}+\mathrm{xsinu}}\mathrm{du}\:=\frac{\pi}{\mathrm{2x}}−\frac{\mathrm{1}}{\mathrm{2x}}\int_{\mathrm{0}} ^{\pi} \:\frac{\mathrm{du}}{\mathrm{1}+\mathrm{xsinu}}\:\mathrm{changement}\:\mathrm{tan}\left(\frac{\mathrm{u}}{\mathrm{2}}\right)=\mathrm{y} \\ $$$$\mathrm{give}\:\int_{\mathrm{0}} ^{\pi} \:\frac{\mathrm{du}}{\mathrm{1}+\mathrm{xsinu}}\:=\int_{\mathrm{0}} ^{\infty} \:\:\frac{\mathrm{2dy}}{\left(\mathrm{1}+\mathrm{y}^{\mathrm{2}} \right)\left(\mathrm{1}+\mathrm{x}\frac{\mathrm{2y}}{\mathrm{1}+\mathrm{y}^{\mathrm{2}} }\right)} \\ $$$$\mathrm{H}=\int_{\mathrm{0}} ^{\infty} \:\:\frac{\mathrm{2dy}}{\mathrm{1}+\mathrm{y}^{\mathrm{2}} \:+\mathrm{2xy}}\:=\int_{\mathrm{0}} ^{\infty} \:\frac{\mathrm{2dy}}{\mathrm{y}^{\mathrm{2}} \:+\mathrm{2xy}\:+\mathrm{1}}\:=\int_{\mathrm{0}} ^{\infty} \:\frac{\mathrm{2dy}}{\left(\mathrm{y}+\mathrm{x}\right)^{\mathrm{2}} \:+\mathrm{1}−\mathrm{x}^{\mathrm{2}} } \\ $$$$\mathrm{case}\:\mathrm{1}\:\:\mathrm{1}−\mathrm{x}^{\mathrm{2}} >\mathrm{0}\:\Rightarrow\mid\mathrm{x}\mid<\mathrm{1}\:\:\Rightarrow\mathrm{H}=_{\mathrm{y}+\mathrm{x}=\sqrt{\mathrm{1}−\mathrm{x}^{\mathrm{2}} }\mathrm{z}} \:\:\:\int_{\frac{\mathrm{x}}{\:\sqrt{\mathrm{1}−\mathrm{x}^{\mathrm{2}} }}} ^{\infty} \:\:\frac{\mathrm{2}\sqrt{\mathrm{1}−\mathrm{x}^{\mathrm{2}} }\mathrm{dz}}{\left(\mathrm{1}−\mathrm{x}^{\mathrm{2}} \right)\left(\mathrm{1}+\mathrm{z}^{\mathrm{2}} \right)} \\ $$$$=\frac{\mathrm{2}}{\:\sqrt{\mathrm{1}−\mathrm{x}^{\mathrm{2}} }}\left[\mathrm{arctanz}\right]_{\frac{\mathrm{x}}{\:\sqrt{\mathrm{1}−\mathrm{x}^{\mathrm{2}} }}} ^{\infty} \:\:=\frac{\mathrm{2}}{\:\sqrt{\mathrm{1}−\mathrm{x}^{\mathrm{2}} }}\left(\frac{\pi}{\mathrm{2}}−\mathrm{arctan}\left(\frac{\mathrm{x}}{\:\sqrt{\mathrm{1}−\mathrm{x}^{\mathrm{2}} }}\right)\right) \\ $$$$=\frac{\pi}{\:\sqrt{\mathrm{1}−\mathrm{x}^{\mathrm{2}} }}−\frac{\mathrm{2}}{\:\sqrt{\mathrm{1}−\mathrm{x}^{\mathrm{2}} }}\mathrm{arctan}\left(\frac{\mathrm{x}}{\:\sqrt{\mathrm{1}−\mathrm{x}^{\mathrm{2}} }}\right)\:\Rightarrow \\ $$$$\mathrm{I}\left(\mathrm{x}\right)=\frac{\pi}{\mathrm{2x}}−\frac{\pi}{\mathrm{2x}\sqrt{\mathrm{1}−\mathrm{x}^{\mathrm{2}} }}−\frac{\mathrm{1}}{\mathrm{x}\sqrt{\mathrm{1}−\mathrm{x}^{\mathrm{2}} }}\mathrm{arctan}\left(\frac{\mathrm{x}}{\:\sqrt{\mathrm{1}−\mathrm{x}^{\mathrm{2}} }}\right) \\ $$$$\mathrm{case}\:\mathrm{2}\:\:\:\mathrm{1}−\mathrm{x}^{\mathrm{2}} <\mathrm{0}\:\:\Rightarrow\mathrm{H}=\int_{\mathrm{0}} ^{\infty} \:\:\:\frac{\mathrm{2dy}}{\left(\mathrm{y}+\mathrm{x}\right)^{\mathrm{2}} −\left(\mathrm{x}^{\mathrm{2}} −\mathrm{1}\right)} \\ $$$$=_{\mathrm{y}+\mathrm{x}=\sqrt{\mathrm{x}^{\mathrm{2}} −\mathrm{1}}\mathrm{u}} \:\:\:\int_{\frac{\mathrm{x}}{\:\sqrt{\mathrm{x}^{\mathrm{2}} −\mathrm{1}}}} ^{\infty} \:\frac{\mathrm{2}\sqrt{\mathrm{x}^{\mathrm{2}} −\mathrm{1}}\mathrm{du}}{\left(\mathrm{x}^{\mathrm{2}} −\mathrm{1}\right)\left(\mathrm{u}^{\mathrm{2}} −\mathrm{1}\right)} \\ $$$$=\frac{\mathrm{2}}{\:\sqrt{\mathrm{x}^{\mathrm{2}} −\mathrm{1}}}\int_{\frac{\mathrm{x}}{\:\sqrt{\mathrm{x}^{\mathrm{2}} −\mathrm{1}}}} ^{\infty} \:\frac{\mathrm{du}}{\left(\mathrm{u}−\mathrm{1}\right)\left(\mathrm{u}+\mathrm{1}\right)} \\ $$$$=\frac{\mathrm{1}}{\:\sqrt{\mathrm{x}^{\mathrm{2}} −\mathrm{1}}}\int_{\frac{\mathrm{x}}{\:\sqrt{\mathrm{x}^{\mathrm{2}} −\mathrm{1}}}} ^{\infty} \:\left(\frac{\mathrm{1}}{\mathrm{u}−\mathrm{1}}−\frac{\mathrm{1}}{\mathrm{u}+\mathrm{1}}\right) \\ $$$$=\frac{\mathrm{1}}{\:\sqrt{\mathrm{x}^{\mathrm{2}} −\mathrm{1}}}\left[\mathrm{log}\mid\frac{\mathrm{u}−\mathrm{1}}{\mathrm{u}+\mathrm{1}}\mid\right]_{\frac{\mathrm{x}}{\:\sqrt{\mathrm{x}^{\mathrm{2}} −\mathrm{1}}}} ^{\infty} \:=−\frac{\mathrm{1}}{\:\sqrt{\mathrm{x}^{\mathrm{2}} −\mathrm{1}}}\mathrm{log}\mid\frac{\frac{\mathrm{x}}{\:\sqrt{\mathrm{x}^{\mathrm{2}} −\mathrm{1}}}−\mathrm{1}}{\frac{\mathrm{x}}{\:\sqrt{\mathrm{x}^{\mathrm{2}} −\mathrm{1}}}+\mathrm{1}}\mid \\ $$$$=−\frac{\mathrm{1}}{\:\sqrt{\mathrm{x}^{\mathrm{2}} −\mathrm{1}}}\mathrm{log}\mid\frac{\mathrm{x}−\sqrt{\mathrm{x}^{\mathrm{2}} −\mathrm{1}}}{\mathrm{x}+\sqrt{\mathrm{x}^{\mathrm{2}} −\mathrm{1}}}\mid\:\Rightarrow \\ $$$$\mathrm{I}\left(\mathrm{x}\right)=\frac{\pi}{\mathrm{2x}}+\frac{\mathrm{1}}{\mathrm{2x}\sqrt{\mathrm{x}^{\mathrm{2}} −\mathrm{1}}}\mathrm{log}\mid\frac{\mathrm{x}−\sqrt{\mathrm{x}^{\mathrm{2}} −\mathrm{1}}}{\mathrm{x}+\sqrt{\mathrm{x}^{\mathrm{2}} −\mathrm{1}}}\mid \\ $$
