
Question Number 166260 by amin96 last updated on 16/Feb/22
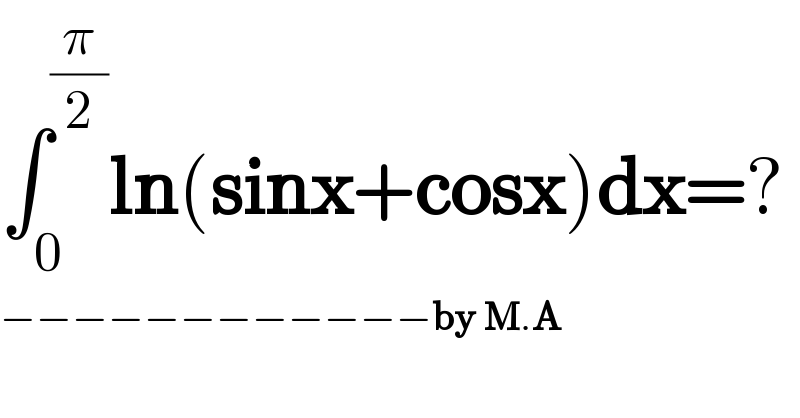
$$\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \boldsymbol{\mathrm{ln}}\left(\boldsymbol{\mathrm{sinx}}+\boldsymbol{\mathrm{cosx}}\right)\boldsymbol{\mathrm{dx}}=? \\ $$$$−−−−−−−−−−−−\boldsymbol{\mathrm{by}}\:\boldsymbol{\mathrm{M}}.\boldsymbol{\mathrm{A}} \\ $$
Answered by Eulerian last updated on 17/Feb/22

$$\: \\ $$$$\:\mathrm{I}\:=\:\int_{\mathrm{0}} ^{\:\frac{\pi}{\mathrm{2}}} \:\mathrm{ln}\left(\mathrm{sin}\:\mathrm{x}\:+\:\mathrm{cos}\:\mathrm{x}\right)\:\mathrm{dx}\:=\:\frac{\mathrm{1}}{\mathrm{2}}\int_{\mathrm{0}} ^{\:\frac{\pi}{\mathrm{2}}} \:\mathrm{ln}\left(\mathrm{1}+\mathrm{sin}\:\mathrm{2x}\right)\:\mathrm{dx} \\ $$$$\:\mathrm{By}\:\mathrm{substitution},\:\mathrm{let}: \\ $$$$\:\mathrm{z}\:=\:\mathrm{2x} \\ $$$$\:\frac{\mathrm{1}}{\mathrm{2}}\:\mathrm{dz}\:=\:\mathrm{dx} \\ $$$$\:\therefore \\ $$$$\:\mathrm{I}\:=\:\frac{\mathrm{1}}{\mathrm{4}}\int_{\mathrm{0}} ^{\:\pi} \:\mathrm{ln}\left(\mathrm{1}+\mathrm{sin}\:\mathrm{z}\right)\:\mathrm{dz}\:=\:\frac{\mathrm{1}}{\mathrm{2}}\int_{\mathrm{0}} ^{\:\frac{\pi}{\mathrm{2}}} \:\mathrm{ln}\left(\mathrm{1}+\mathrm{sin}\:\mathrm{z}\right)\:\mathrm{dz} \\ $$$$\: \\ $$$$\:\mathrm{By}\:\mathrm{noticing}\:\mathrm{that}\:\mathrm{it}\:\mathrm{somehow}\:\mathrm{looks}\:\mathrm{like}\:\mathrm{the}\:\mathrm{integral}\:\mathrm{representation}\:\mathrm{of}\:\mathrm{Catalan}'\mathrm{s} \\ $$$$\:\mathrm{constant},\:\mathrm{such}\:\mathrm{that}: \\ $$$$\:\mathrm{G}\:=\:\frac{\mathrm{1}}{\mathrm{2}}\int_{\mathrm{0}} ^{\:\frac{\pi}{\mathrm{2}}} \:\mathrm{ln}\left(\mathrm{sec}\:\mathrm{z}\:+\:\mathrm{tan}\:\mathrm{z}\right)\:\mathrm{dz}\:=\:\frac{\mathrm{1}}{\mathrm{2}}\int_{\mathrm{0}} ^{\:\frac{\pi}{\mathrm{2}}} \:\mathrm{ln}\left(\mathrm{1}\:+\:\mathrm{sin}\:\mathrm{z}\right)\:−\:\mathrm{ln}\left(\mathrm{cos}\:\mathrm{z}\right)\:\mathrm{dz} \\ $$$$\: \\ $$$$\:\therefore \\ $$$$\:\mathrm{I}\:=\:\frac{\mathrm{1}}{\mathrm{2}}\int_{\mathrm{0}} ^{\:\frac{\pi}{\mathrm{2}}} \:\mathrm{ln}\left(\mathrm{1}+\mathrm{sin}\:\mathrm{z}\right)\:−\:\mathrm{ln}\left(\mathrm{cos}\:\mathrm{z}\right)\:+\:\mathrm{ln}\left(\mathrm{cos}\:\mathrm{z}\right)\:\mathrm{dz} \\ $$$$\:\:\:\:=\:\frac{\mathrm{1}}{\mathrm{2}}\int_{\mathrm{0}} ^{\:\frac{\pi}{\mathrm{2}}} \mathrm{ln}\left(\mathrm{1}+\mathrm{sin}\:\mathrm{z}\right)\:−\:\mathrm{ln}\left(\mathrm{cos}\:\mathrm{z}\right)\:\mathrm{dz}\:+\:\frac{\mathrm{1}}{\mathrm{2}}\int_{\mathrm{0}} ^{\:\frac{\pi}{\mathrm{2}}} \mathrm{ln}\left(\mathrm{cos}\:\mathrm{z}\right)\:\mathrm{dz} \\ $$$$\:\:\:\:=\:\mathrm{G}\:+\:\frac{\mathrm{1}}{\mathrm{2}}\int_{\mathrm{0}} ^{\:\frac{\pi}{\mathrm{2}}} \mathrm{ln}\left(\mathrm{sin}\:\mathrm{z}\right)\:\mathrm{dz}\:\:\:\:\:\:\:\left(\boldsymbol{\mathrm{King}}\:\boldsymbol{\mathrm{Rule}}\right) \\ $$$$\: \\ $$$$\:\mathrm{By}\:\mathrm{adding}\:\mathrm{those}\:\mathrm{integrals},\:\mathrm{we}\:\mathrm{get}: \\ $$$$\:\mathrm{2I}\:=\:\mathrm{2G}\:+\:\frac{\mathrm{1}}{\mathrm{2}}\int_{\mathrm{0}} ^{\:\frac{\pi}{\mathrm{2}}} \mathrm{ln}\left(\mathrm{sin}\:\mathrm{z}\right)\:\mathrm{dz}\:+\:\frac{\mathrm{1}}{\mathrm{2}}\int_{\mathrm{0}} ^{\:\frac{\pi}{\mathrm{2}}} \mathrm{ln}\left(\mathrm{cos}\:\mathrm{z}\right)\:\mathrm{dz} \\ $$$$\:\mathrm{I}\:=\:\mathrm{G}\:+\:\frac{\mathrm{1}}{\mathrm{4}}\int_{\mathrm{0}} ^{\:\frac{\pi}{\mathrm{2}}} \mathrm{ln}\left(\mathrm{sin}\:\mathrm{2z}\right)\:−\:\mathrm{ln}\left(\mathrm{2}\right)\:\mathrm{dz} \\ $$$$\:\:\:\:=\:\mathrm{G}\:+\:\frac{\mathrm{1}}{\mathrm{4}}\int_{\mathrm{0}} ^{\:\frac{\pi}{\mathrm{2}}} \mathrm{ln}\left(\mathrm{sin}\:\mathrm{2z}\right)\:\mathrm{dz}\:−\:\frac{\mathrm{1}}{\mathrm{4}}\int_{\mathrm{0}} ^{\:\frac{\pi}{\mathrm{2}}} \mathrm{ln}\left(\mathrm{2}\right)\:\mathrm{dz} \\ $$$$\:\:\:\:=\:\mathrm{G}\:+\:\frac{\mathrm{1}}{\mathrm{8}}\int_{\mathrm{0}} ^{\:\pi} \mathrm{ln}\left(\mathrm{sin}\:\mathrm{z}\right)\:\mathrm{dz}\:−\:\frac{\pi\mathrm{ln}\left(\mathrm{2}\right)}{\mathrm{8}} \\ $$$$\:\:\:\:=\:\mathrm{G}\:−\:\frac{\pi\mathrm{ln}\left(\mathrm{2}\right)}{\mathrm{8}}\:+\:\frac{\mathrm{1}}{\mathrm{4}}\int_{\mathrm{0}} ^{\:\frac{\pi}{\mathrm{2}}} \mathrm{ln}\left(\mathrm{sin}\:\mathrm{z}\right)\:\mathrm{dz} \\ $$$$\:\: \\ $$$$\:\mathrm{From}\:\mathrm{what}\:\mathrm{we}\:\mathrm{have}\:\mathrm{earlier},\:\mathrm{by}\:\mathrm{comparing}\:\mathrm{to}\:\mathrm{what}\:\mathrm{we}\:\mathrm{have}\:\mathrm{right}\:\mathrm{now},\:\mathrm{we}\:\mathrm{get}: \\ $$$$\:\mathrm{G}\:+\:\frac{\mathrm{1}}{\mathrm{2}}\int_{\mathrm{0}} ^{\:\frac{\pi}{\mathrm{2}}} \mathrm{ln}\left(\mathrm{sin}\:\mathrm{z}\right)\:\mathrm{dz}\:=\:\mathrm{G}\:−\:\frac{\pi\mathrm{ln}\left(\mathrm{2}\right)}{\mathrm{8}}\:+\:\frac{\mathrm{1}}{\mathrm{4}}\int_{\mathrm{0}} ^{\:\frac{\pi}{\mathrm{2}}} \mathrm{ln}\left(\mathrm{sin}\:\mathrm{z}\right)\:\mathrm{dz} \\ $$$$\:\frac{\mathrm{1}}{\mathrm{2}}\int_{\mathrm{0}} ^{\:\frac{\pi}{\mathrm{2}}} \mathrm{ln}\left(\mathrm{sin}\:\mathrm{z}\right)\:\mathrm{dz}\:=\:−\frac{\pi\mathrm{ln}\left(\mathrm{2}\right)}{\mathrm{8}}\:+\:\frac{\mathrm{1}}{\mathrm{4}}\int_{\mathrm{0}} ^{\:\frac{\pi}{\mathrm{2}}} \mathrm{ln}\left(\mathrm{sin}\:\mathrm{z}\right)\:\mathrm{dz} \\ $$$$\:\frac{\mathrm{1}}{\mathrm{2}}\int_{\mathrm{0}} ^{\:\frac{\pi}{\mathrm{2}}} \mathrm{ln}\left(\mathrm{sin}\:\mathrm{z}\right)\:\mathrm{dz}\:−\:\frac{\mathrm{1}}{\mathrm{4}}\int_{\mathrm{0}} ^{\:\frac{\pi}{\mathrm{2}}} \mathrm{ln}\left(\mathrm{sin}\:\mathrm{z}\right)\:\mathrm{dz}\:=\:−\frac{\pi\mathrm{ln}\left(\mathrm{2}\right)}{\mathrm{8}} \\ $$$$\:\int_{\mathrm{0}} ^{\:\frac{\pi}{\mathrm{2}}} \mathrm{ln}\left(\mathrm{sin}\:\mathrm{z}\right)\:\mathrm{dz}\:=\:−\frac{\pi\mathrm{ln}\left(\mathrm{2}\right)}{\mathrm{2}} \\ $$$$\: \\ $$$$\:\mathrm{Finally},\:\mathrm{we}\:\mathrm{now}\:\mathrm{have}\:\mathrm{our}\:\mathrm{answer}\:\mathrm{to}\:\mathrm{integral}\:\mathrm{I}: \\ $$$$\:\mathrm{I}\:=\:\mathrm{G}\:−\:\frac{\pi\mathrm{ln}\left(\mathrm{2}\right)}{\mathrm{8}}\:−\:\left(\frac{\mathrm{1}}{\mathrm{4}}\right)\centerdot\frac{\pi\mathrm{ln}\left(\mathrm{2}\right)}{\mathrm{2}}\:=\:\mathrm{G}\:−\:\frac{\pi\mathrm{ln}\left(\mathrm{2}\right)}{\mathrm{4}} \\ $$$$\: \\ $$$$\:\boldsymbol{\mathrm{Solution}}\:\boldsymbol{\mathrm{by}}:\:\mathrm{Kevin} \\ $$
Commented by amin96 last updated on 17/Feb/22

$$\boldsymbol{\mathrm{correct}}\:\boldsymbol{\mathrm{answer}}\:\boldsymbol{\mathrm{bravo}}\:\boldsymbol{\mathrm{sir}}\:\boldsymbol{\mathrm{Kevin}} \\ $$
