
Question Number 98678 by PRITHWISH SEN 2 last updated on 15/Jun/20

$$\int_{\mathrm{0}} ^{\infty} \boldsymbol{\mathrm{e}}^{−\boldsymbol{\mathrm{ax}}} \frac{\mathrm{sin}\:\boldsymbol{\mathrm{mx}}}{\boldsymbol{\mathrm{x}}}\:\boldsymbol{\mathrm{dx}}\:=\:\mathrm{tan}^{−\mathrm{1}} \left(\frac{\boldsymbol{\mathrm{m}}}{\boldsymbol{\mathrm{a}}}\right),\:\boldsymbol{\mathrm{a}}>\mathrm{0} \\ $$
Commented byTinku Tara last updated on 15/Jun/20

$$\mathrm{Are}\:\mathrm{you}\:\mathrm{facing}\:\mathrm{problem}\:\mathrm{with} \\ $$ $$\mathrm{2}.\mathrm{083}?\:\mathrm{please}\:\mathrm{let}\:\mathrm{us}\:\mathrm{know}.\:\mathrm{if} \\ $$ $$\mathrm{there}\:\mathrm{is}\:\mathrm{crash}\:\mathrm{please}\:\mathrm{report}. \\ $$
Commented byPRITHWISH SEN 2 last updated on 15/Jun/20

$$\mathrm{actually}\:\mathrm{it}\:\mathrm{is}\:\mathrm{not}\:\mathrm{opening}\:\mathrm{for}\:\mathrm{most}\:\mathrm{of}\:\mathrm{the}\:\mathrm{time}. \\ $$
Commented byTinku Tara last updated on 15/Jun/20
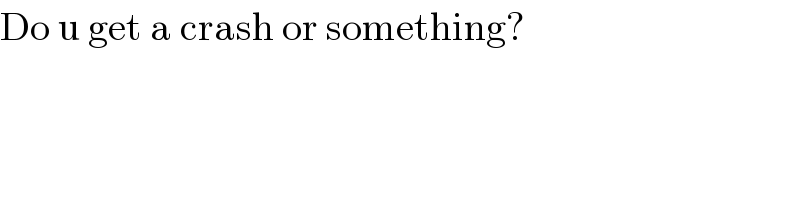
$$\mathrm{Do}\:\mathrm{u}\:\mathrm{get}\:\mathrm{a}\:\mathrm{crash}\:\mathrm{or}\:\mathrm{something}? \\ $$
Commented byTinku Tara last updated on 15/Jun/20
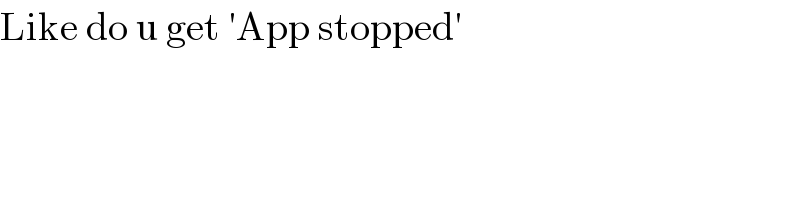
$$\mathrm{Like}\:\mathrm{do}\:\mathrm{u}\:\mathrm{get}\:'\mathrm{App}\:\mathrm{stopped}' \\ $$
Commented byRasheed.Sindhi last updated on 15/Jun/20

$${Where}\:{is}\:{this}\:{version}? \\ $$ $${www}.{tinkutara}.{com}\:{offers} \\ $$ $$\mathrm{2}.\mathrm{081}\:{as}\:{lattest}\:{version}! \\ $$
Commented byTinku Tara last updated on 15/Jun/20

$$\mathrm{Playstore}\:\mathrm{and}\:\mathrm{tinkutara}.\mathrm{com} \\ $$ $$\mathrm{both}\:\mathrm{have}\:\mathrm{2}.\mathrm{083}.\:\mathrm{2}.\mathrm{083}\:\mathrm{introduced} \\ $$ $$\mathrm{background}\:\mathrm{color}\:\mathrm{and}\:\mathrm{more}\:\mathrm{font} \\ $$ $$\mathrm{color}. \\ $$
Commented byRasheed.Sindhi last updated on 15/Jun/20

$$\mathcal{H}{ow}\:{to}\:{change}\:{background}\:{sir}? \\ $$
Commented byTinku Tara last updated on 15/Jun/20

$$\mathrm{only}\:\mathrm{available}\:\mathrm{in}\:\mathrm{v2}.\mathrm{083}\:\mathrm{in}\:\mathrm{offline} \\ $$ $$\mathrm{editor}. \\ $$
Commented byPRITHWISH SEN 2 last updated on 16/Jun/20

$$\mathrm{I}\:\mathrm{have}\:\mathrm{updated}\:\mathrm{2}.\mathrm{084}\:\mathrm{version}.\:\mathrm{It}\:\mathrm{is}\:\mathrm{opening}\:\mathrm{but}\:\mathrm{it} \\ $$ $$\mathrm{is}\:\mathrm{taking}\:\mathrm{some}\:\mathrm{time}. \\ $$
Answered by mathmax by abdo last updated on 15/Jun/20
![let f(a) =∫_0 ^∞ e^(−ax) ((sin(mx))/x) dx ⇒f^′ (a) =−∫_0 ^∞ e^(−ax) sin(mx)dx =−Im(∫_0 ^∞ e^(−ax+imx) dx) we have ∫_0 ^∞ e^((−a+im)x) dx =[(1/(−a+im)) e^((−a+im)x) ]_0 ^∞ =−(1/(a−im))(−1) =(1/(a−im)) =((a+im)/(a^2 +m^2 )) ⇒f^′ (a) =−(m/(a^2 +m^2 )) ⇒ f(a) =−m∫ (da/(a^2 +m^2 )) +c (a=mu) =−m∫ ((mdu)/(m^2 (1+u^2 ))) =−∫ (du/(1+u^2 )) =−arctan((a/m))+c (we suppoze m≠0) if m>0 we get lim_(a→+∞) f(a) =0 =−(π/2) +c ⇒c =(π/2) ⇒ f(a) =(π/2) −arctan((a/m)) =arctan((m/a)) if m<0 we get lim_(a→+∞) f(a) =0 =(π/2) +c ⇒c =−(π/2) ⇒ f(a) =−arctan((a/m))−(π/2) =−((π/2) +arctam((a/m))) =−((π/2)−arctan(−(a/m))) =−arctan(−(m/a)) = arctan((m/a)) so in all case we have f(a) =arctan((m/a)) if m=0 ⇒f(a) =0](Q98685.png)
$$\mathrm{let}\:\mathrm{f}\left(\mathrm{a}\right)\:=\int_{\mathrm{0}} ^{\infty} \:\mathrm{e}^{−\mathrm{ax}} \:\frac{\mathrm{sin}\left(\mathrm{mx}\right)}{\mathrm{x}}\:\mathrm{dx}\:\Rightarrow\mathrm{f}^{'} \left(\mathrm{a}\right)\:=−\int_{\mathrm{0}} ^{\infty} \:\mathrm{e}^{−\mathrm{ax}} \:\mathrm{sin}\left(\mathrm{mx}\right)\mathrm{dx} \\ $$ $$=−\mathrm{Im}\left(\int_{\mathrm{0}} ^{\infty} \:\mathrm{e}^{−\mathrm{ax}+\mathrm{imx}} \mathrm{dx}\right)\:\:\mathrm{we}\:\mathrm{have}\:\int_{\mathrm{0}} ^{\infty} \:\mathrm{e}^{\left(−\mathrm{a}+\mathrm{im}\right)\mathrm{x}} \:\mathrm{dx}\:=\left[\frac{\mathrm{1}}{−\mathrm{a}+\mathrm{im}}\:\mathrm{e}^{\left(−\mathrm{a}+\mathrm{im}\right)\mathrm{x}} \right]_{\mathrm{0}} ^{\infty} \\ $$ $$=−\frac{\mathrm{1}}{\mathrm{a}−\mathrm{im}}\left(−\mathrm{1}\right)\:=\frac{\mathrm{1}}{\mathrm{a}−\mathrm{im}}\:=\frac{\mathrm{a}+\mathrm{im}}{\mathrm{a}^{\mathrm{2}} \:+\mathrm{m}^{\mathrm{2}} }\:\Rightarrow\mathrm{f}^{'} \left(\mathrm{a}\right)\:=−\frac{\mathrm{m}}{\mathrm{a}^{\mathrm{2}} \:+\mathrm{m}^{\mathrm{2}} }\:\Rightarrow \\ $$ $$\mathrm{f}\left(\mathrm{a}\right)\:=−\mathrm{m}\int\:\:\frac{\mathrm{da}}{\mathrm{a}^{\mathrm{2}} \:+\mathrm{m}^{\mathrm{2}} }\:+\mathrm{c}\:\:\:\left(\mathrm{a}=\mathrm{mu}\right) \\ $$ $$=−\mathrm{m}\int\:\:\:\frac{\mathrm{mdu}}{\mathrm{m}^{\mathrm{2}} \left(\mathrm{1}+\mathrm{u}^{\mathrm{2}} \right)}\:=−\int\:\frac{\mathrm{du}}{\mathrm{1}+\mathrm{u}^{\mathrm{2}} }\:=−\mathrm{arctan}\left(\frac{\mathrm{a}}{\mathrm{m}}\right)+\mathrm{c}\:\:\:\left(\mathrm{we}\:\mathrm{suppoze}\:\mathrm{m}\neq\mathrm{0}\right) \\ $$ $$\mathrm{if}\:\mathrm{m}>\mathrm{0}\:\mathrm{we}\:\mathrm{get}\:\mathrm{lim}_{\mathrm{a}\rightarrow+\infty} \mathrm{f}\left(\mathrm{a}\right)\:=\mathrm{0}\:=−\frac{\pi}{\mathrm{2}}\:+\mathrm{c}\:\Rightarrow\mathrm{c}\:=\frac{\pi}{\mathrm{2}}\:\Rightarrow \\ $$ $$\mathrm{f}\left(\mathrm{a}\right)\:=\frac{\pi}{\mathrm{2}}\:−\mathrm{arctan}\left(\frac{\mathrm{a}}{\mathrm{m}}\right)\:=\mathrm{arctan}\left(\frac{\mathrm{m}}{\mathrm{a}}\right) \\ $$ $$\mathrm{if}\:\mathrm{m}<\mathrm{0}\:\mathrm{we}\:\mathrm{get}\:\mathrm{lim}_{\mathrm{a}\rightarrow+\infty} \mathrm{f}\left(\mathrm{a}\right)\:=\mathrm{0}\:=\frac{\pi}{\mathrm{2}}\:+\mathrm{c}\:\Rightarrow\mathrm{c}\:=−\frac{\pi}{\mathrm{2}}\:\Rightarrow \\ $$ $$\mathrm{f}\left(\mathrm{a}\right)\:=−\mathrm{arctan}\left(\frac{\mathrm{a}}{\mathrm{m}}\right)−\frac{\pi}{\mathrm{2}}\:=−\left(\frac{\pi}{\mathrm{2}}\:+\mathrm{arctam}\left(\frac{\mathrm{a}}{\mathrm{m}}\right)\right) \\ $$ $$=−\left(\frac{\pi}{\mathrm{2}}−\mathrm{arctan}\left(−\frac{\mathrm{a}}{\mathrm{m}}\right)\right)\:=−\mathrm{arctan}\left(−\frac{\mathrm{m}}{\mathrm{a}}\right)\:=\:\mathrm{arctan}\left(\frac{\mathrm{m}}{\mathrm{a}}\right)\:\mathrm{so}\:\mathrm{in}\:\mathrm{all}\:\mathrm{case} \\ $$ $$\mathrm{we}\:\mathrm{have}\:\mathrm{f}\left(\mathrm{a}\right)\:=\mathrm{arctan}\left(\frac{\mathrm{m}}{\mathrm{a}}\right)\: \\ $$ $$\mathrm{if}\:\mathrm{m}=\mathrm{0}\:\:\Rightarrow\mathrm{f}\left(\mathrm{a}\right)\:=\mathrm{0} \\ $$ $$ \\ $$
Commented byPRITHWISH SEN 2 last updated on 15/Jun/20

$$\mathrm{thank}\:\mathrm{you}\:\mathrm{sir} \\ $$
Commented bymathmax by abdo last updated on 15/Jun/20

$$\mathrm{you}\:\mathrm{are}\:\mathrm{welcome} \\ $$
Answered by smridha last updated on 15/Jun/20
![let′s do it by Laplace transform f(s)=L[sin(mx)]=∫_0 ^∞ e^(−sx) sin(mx)dx =(m/(s^2 +m^2 )) now L[((sin(mx))/x)]=∫_a ^∞ (m/(s^2 +m^2 ))ds =m.(1/m)[tan^(−1) ((s/m))]_a ^∞ =(π/2)−tan^(−1) ((a/m))=cot^(−1) ((a/m))=tan^(−1) ((m/a))where a>0](Q98724.png)
$$\boldsymbol{{let}}'{s}\:\boldsymbol{{do}}\:\boldsymbol{{it}}\:\boldsymbol{{by}}\:\boldsymbol{{L}}{aplace}\:\boldsymbol{{transform}} \\ $$ $$\boldsymbol{{f}}\left(\boldsymbol{{s}}\right)=\boldsymbol{{L}}\left[\boldsymbol{{sin}}\left(\boldsymbol{{mx}}\right)\right]=\int_{\mathrm{0}} ^{\infty} \boldsymbol{{e}}^{−\boldsymbol{{sx}}} \boldsymbol{{sin}}\left(\boldsymbol{{mx}}\right)\boldsymbol{{dx}} \\ $$ $$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\frac{\boldsymbol{{m}}}{\boldsymbol{{s}}^{\mathrm{2}} +\boldsymbol{{m}}^{\mathrm{2}} } \\ $$ $$\boldsymbol{{now}}\:\boldsymbol{{L}}\left[\frac{\boldsymbol{{sin}}\left(\boldsymbol{{mx}}\right)}{\boldsymbol{{x}}}\right]=\int_{\boldsymbol{{a}}} ^{\infty} \frac{\boldsymbol{{m}}}{\boldsymbol{{s}}^{\mathrm{2}} +\boldsymbol{{m}}^{\mathrm{2}} }\boldsymbol{{ds}} \\ $$ $$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\boldsymbol{{m}}.\frac{\mathrm{1}}{\boldsymbol{{m}}}\left[\mathrm{tan}^{−\mathrm{1}} \left(\frac{\boldsymbol{{s}}}{\boldsymbol{{m}}}\right)\right]_{\boldsymbol{{a}}} ^{\infty} \\ $$ $$=\frac{\pi}{\mathrm{2}}−\mathrm{tan}^{−\mathrm{1}} \left(\frac{\boldsymbol{{a}}}{\boldsymbol{{m}}}\right)=\mathrm{cot}^{−\mathrm{1}} \left(\frac{\boldsymbol{{a}}}{\boldsymbol{{m}}}\right)=\mathrm{tan}^{−\mathrm{1}} \left(\frac{\boldsymbol{{m}}}{\boldsymbol{{a}}}\right)\boldsymbol{{where}}\:\boldsymbol{{a}}>\mathrm{0} \\ $$
Commented byPRITHWISH SEN 2 last updated on 16/Jun/20

$$\mathrm{thank}\:\mathrm{you}\:\mathrm{sir} \\ $$
Commented bysmridha last updated on 16/Jun/20

$$\boldsymbol{{welcome}} \\ $$
