Question and Answers Forum

All Questions      Topic List

None Questions

Previous in All Question      Next in All Question      

Previous in None      Next in None      

Question Number 135689 by joki last updated on 15/Mar/21

0,....,....,(5/4),(4/5),.... how to solution aritmatic

$$\mathrm{0},....,....,\frac{\mathrm{5}}{\mathrm{4}},\frac{\mathrm{4}}{\mathrm{5}},.... \\ $$$$\mathrm{how}\:\mathrm{to}\:\mathrm{solution}\:\mathrm{aritmatic} \\ $$

Commented by liberty last updated on 15/Mar/21

AP ?

$${AP}\:? \\ $$

Commented by joki last updated on 15/Mar/21

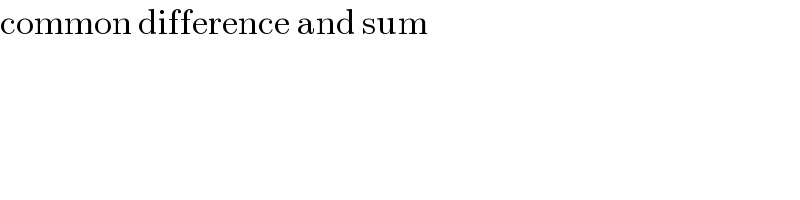
common difference and sum

$$\mathrm{common}\:\mathrm{difference}\:\mathrm{and}\:\mathrm{sum}\: \\ $$$$ \\ $$

Commented by mr W last updated on 15/Mar/21

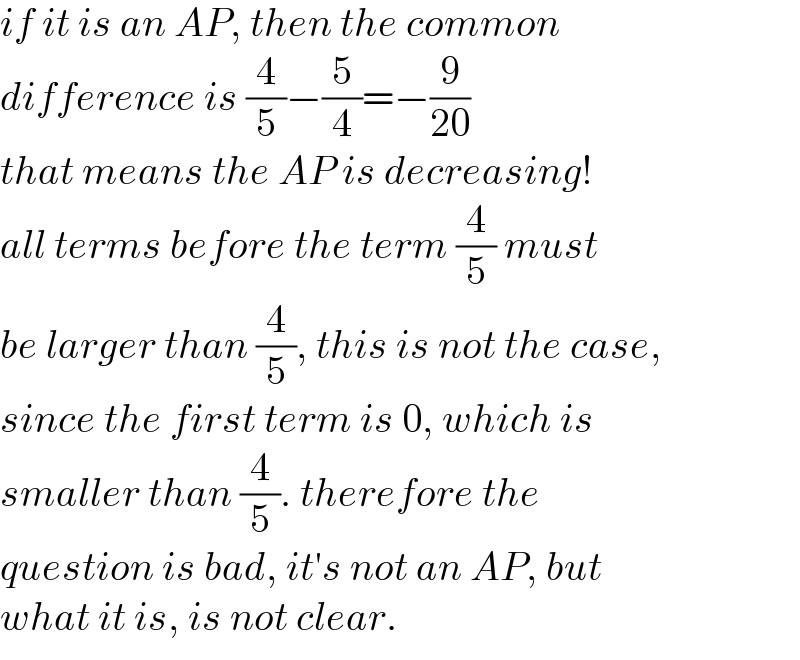
if it is an AP, then the common difference is (4/5)−(5/4)=−(9/(20)) that means the AP is decreasing! all terms before the term (4/5) must be larger than (4/5), this is not the case, since the first term is 0, which is smaller than (4/5). therefore the question is bad, it′s not an AP, but what it is, is not clear.

$${if}\:{it}\:{is}\:{an}\:{AP},\:{then}\:{the}\:{common} \\ $$$${difference}\:{is}\:\frac{\mathrm{4}}{\mathrm{5}}−\frac{\mathrm{5}}{\mathrm{4}}=−\frac{\mathrm{9}}{\mathrm{20}} \\ $$$${that}\:{means}\:{the}\:{AP}\:{is}\:{decreasing}! \\ $$$${all}\:{terms}\:{before}\:{the}\:{term}\:\frac{\mathrm{4}}{\mathrm{5}}\:{must} \\ $$$${be}\:{larger}\:{than}\:\frac{\mathrm{4}}{\mathrm{5}},\:{this}\:{is}\:{not}\:{the}\:{case}, \\ $$$${since}\:{the}\:{first}\:{term}\:{is}\:\mathrm{0},\:{which}\:{is} \\ $$$${smaller}\:{than}\:\frac{\mathrm{4}}{\mathrm{5}}.\:{therefore}\:{the} \\ $$$${question}\:{is}\:{bad},\:{it}'{s}\:{not}\:{an}\:{AP},\:{but} \\ $$$${what}\:{it}\:{is},\:{is}\:{not}\:{clear}. \\ $$

Commented by MJS_new last updated on 15/Mar/21

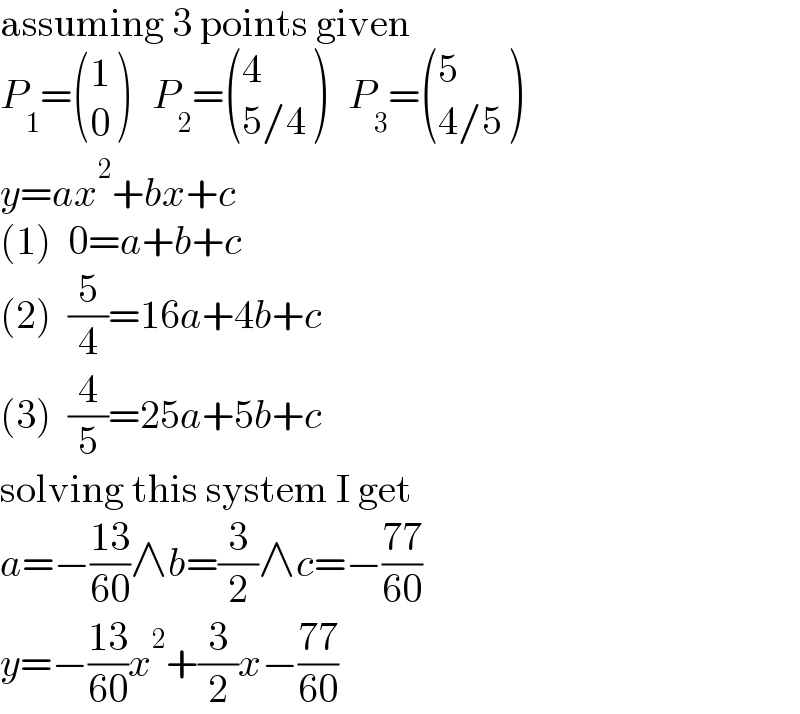
assuming 3 points given P_1 = ((1),(0) ) P_2 = ((4),((5/4)) ) P_3 = ((5),((4/5)) ) y=ax^2 +bx+c (1) 0=a+b+c (2) (5/4)=16a+4b+c (3) (4/5)=25a+5b+c solving this system I get a=−((13)/(60))∧b=(3/2)∧c=−((77)/(60)) y=−((13)/(60))x^2 +(3/2)x−((77)/(60))

$$\mathrm{assuming}\:\mathrm{3}\:\mathrm{points}\:\mathrm{given} \\ $$$${P}_{\mathrm{1}} =\begin{pmatrix}{\mathrm{1}}\\{\mathrm{0}}\end{pmatrix}\:\:\:{P}_{\mathrm{2}} =\begin{pmatrix}{\mathrm{4}}\\{\mathrm{5}/\mathrm{4}}\end{pmatrix}\:\:\:{P}_{\mathrm{3}} =\begin{pmatrix}{\mathrm{5}}\\{\mathrm{4}/\mathrm{5}}\end{pmatrix} \\ $$$${y}={ax}^{\mathrm{2}} +{bx}+{c} \\ $$$$\left(\mathrm{1}\right)\:\:\mathrm{0}={a}+{b}+{c} \\ $$$$\left(\mathrm{2}\right)\:\:\frac{\mathrm{5}}{\mathrm{4}}=\mathrm{16}{a}+\mathrm{4}{b}+{c} \\ $$$$\left(\mathrm{3}\right)\:\:\frac{\mathrm{4}}{\mathrm{5}}=\mathrm{25}{a}+\mathrm{5}{b}+{c} \\ $$$$\mathrm{solving}\:\mathrm{this}\:\mathrm{system}\:\mathrm{I}\:\mathrm{get} \\ $$$${a}=−\frac{\mathrm{13}}{\mathrm{60}}\wedge{b}=\frac{\mathrm{3}}{\mathrm{2}}\wedge{c}=−\frac{\mathrm{77}}{\mathrm{60}} \\ $$$${y}=−\frac{\mathrm{13}}{\mathrm{60}}{x}^{\mathrm{2}} +\frac{\mathrm{3}}{\mathrm{2}}{x}−\frac{\mathrm{77}}{\mathrm{60}} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com