Question and Answers Forum

All Questions      Topic List

Integration Questions

Previous in All Question      Next in All Question      

Previous in Integration      Next in Integration      

Question Number 37404 by ajfour last updated on 12/Jun/18

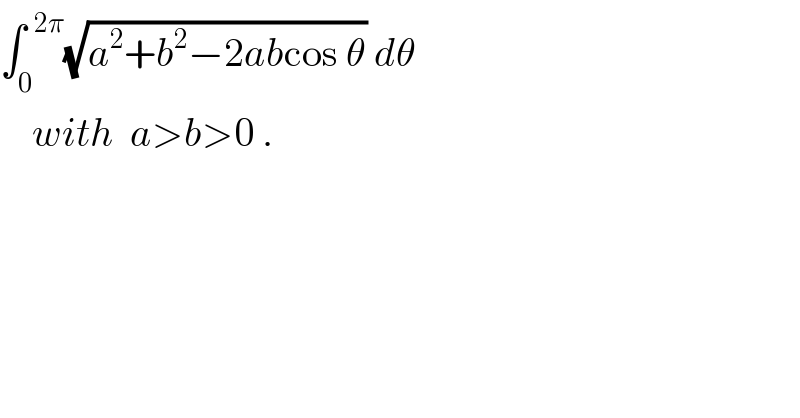
∫_0 ^( 2π) (√(a^2 +b^2 −2abcos θ)) dθ with a>b>0 .

$$\int_{\mathrm{0}} ^{\:\:\mathrm{2}\pi} \sqrt{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} −\mathrm{2}{ab}\mathrm{cos}\:\theta}\:{d}\theta\: \\ $$ $$\:\:\:\:{with}\:\:{a}>{b}>\mathrm{0}\:. \\ $$

Answered by tanmay.chaudhury50@gmail.com last updated on 13/Jun/18

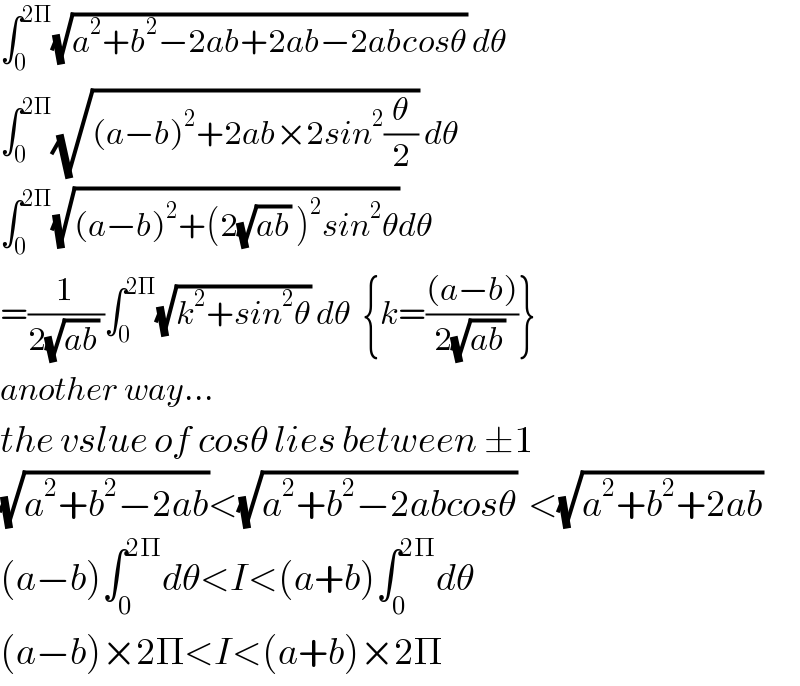
∫_0 ^(2Π) (√(a^2 +b^2 −2ab+2ab−2abcosθ)) dθ ∫_0 ^(2Π) (√((a−b)^2 +2ab×2sin^2 (θ/2))) dθ ∫_0 ^(2Π) (√((a−b)^2 +(2(√(ab)) )^2 sin^2 θ))dθ =(1/(2(√(ab)) ))∫_0 ^(2Π) (√(k^2 +sin^2 θ)) dθ {k=(((a−b))/(2(√(ab)) ))} another way... the vslue of cosθ lies between ±1 (√(a^2 +b^2 −2ab))<(√(a^2 +b^2 −2abcosθ)) <(√(a^2 +b^2 +2ab)) (a−b)∫_0 ^(2Π) dθ<I<(a+b)∫_0 ^(2Π) dθ (a−b)×2Π<I<(a+b)×2Π

$$\int_{\mathrm{0}} ^{\mathrm{2}\Pi} \sqrt{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} −\mathrm{2}{ab}+\mathrm{2}{ab}−\mathrm{2}{abcos}\theta}\:{d}\theta \\ $$ $$\int_{\mathrm{0}} ^{\mathrm{2}\Pi} \sqrt{\left({a}−{b}\right)^{\mathrm{2}} +\mathrm{2}{ab}×\mathrm{2}{sin}^{\mathrm{2}} \frac{\theta}{\mathrm{2}}}\:{d}\theta \\ $$ $$\int_{\mathrm{0}} ^{\mathrm{2}\Pi} \sqrt{\left({a}−{b}\right)^{\mathrm{2}} +\left(\mathrm{2}\sqrt{{ab}}\:\right)^{\mathrm{2}} {sin}^{\mathrm{2}} \theta}{d}\theta \\ $$ $$=\frac{\mathrm{1}}{\mathrm{2}\sqrt{{ab}}\:}\int_{\mathrm{0}} ^{\mathrm{2}\Pi} \sqrt{{k}^{\mathrm{2}} +{sin}^{\mathrm{2}} \theta}\:{d}\theta\:\:\left\{{k}=\frac{\left({a}−{b}\right)}{\mathrm{2}\sqrt{{ab}}\:}\right\} \\ $$ $${another}\:{way}... \\ $$ $${the}\:{vslue}\:{of}\:{cos}\theta\:{lies}\:{between}\:\pm\mathrm{1} \\ $$ $$\sqrt{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} −\mathrm{2}{ab}}<\sqrt{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} −\mathrm{2}{abcos}\theta}\:\:<\sqrt{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} +\mathrm{2}{ab}} \\ $$ $$\left({a}−{b}\right)\int_{\mathrm{0}} ^{\mathrm{2}\Pi} {d}\theta<{I}<\left({a}+{b}\right)\int_{\mathrm{0}} ^{\mathrm{2}\Pi} {d}\theta \\ $$ $$\left({a}−{b}\right)×\mathrm{2}\Pi<{I}<\left({a}+{b}\right)×\mathrm{2}\Pi \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com