
Question Number 152088 by rexford last updated on 25/Aug/21
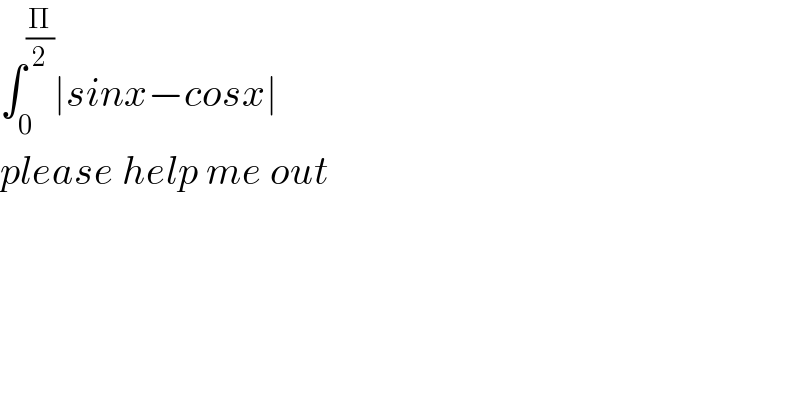
$$\int_{\mathrm{0}} ^{\frac{\Pi}{\mathrm{2}}} \mid{sinx}−{cosx}\mid \\ $$$${please}\:{help}\:{me}\:{out} \\ $$
Answered by Olaf_Thorendsen last updated on 25/Aug/21
![I = ∫_0 ^(π/2) ∣sinx−cosx∣ dx I = ∫_0 ^(π/2) ∣(√2)sin((π/4)−x)∣ dx I = 2∫_0 ^(π/4) (√2)sin((π/4)−x) dx I = 2(√2)[cos((π/4)−x)]_0 ^(π/4) I = 2(√2)(1−(1/( (√2)))) = 2((√2)−1)](Q152092.png)
$$\mathrm{I}\:=\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \mid\mathrm{sin}{x}−\mathrm{cos}{x}\mid\:{dx} \\ $$$$\mathrm{I}\:=\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \mid\sqrt{\mathrm{2}}\mathrm{sin}\left(\frac{\pi}{\mathrm{4}}−{x}\right)\mid\:{dx} \\ $$$$\mathrm{I}\:=\:\mathrm{2}\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \sqrt{\mathrm{2}}\mathrm{sin}\left(\frac{\pi}{\mathrm{4}}−{x}\right)\:{dx} \\ $$$$\mathrm{I}\:=\:\mathrm{2}\sqrt{\mathrm{2}}\left[\mathrm{cos}\left(\frac{\pi}{\mathrm{4}}−{x}\right)\right]_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \\ $$$$\mathrm{I}\:=\:\mathrm{2}\sqrt{\mathrm{2}}\left(\mathrm{1}−\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}\right)\:=\:\mathrm{2}\left(\sqrt{\mathrm{2}}−\mathrm{1}\right) \\ $$
Answered by puissant last updated on 25/Aug/21
![=∫_0 ^(π/4) (cosx−sinx)dx+∫_(π/4) ^(π/2) (sinx−cosx)dx =[sinx+cosx]_0 ^(π/4) +[−cosx−sinx]_(π/4) ^(π/2) = (√2)−1+(−1+(√2)) =2(√2)−2 =2((√2)−1)](Q152093.png)
$$=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \left({cosx}−{sinx}\right){dx}+\int_{\frac{\pi}{\mathrm{4}}} ^{\frac{\pi}{\mathrm{2}}} \left({sinx}−{cosx}\right){dx} \\ $$$$=\left[{sinx}+{cosx}\right]_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} +\left[−{cosx}−{sinx}\right]_{\frac{\pi}{\mathrm{4}}} ^{\frac{\pi}{\mathrm{2}}} \\ $$$$=\:\sqrt{\mathrm{2}}−\mathrm{1}+\left(−\mathrm{1}+\sqrt{\mathrm{2}}\right) \\ $$$$ \\ $$$$=\mathrm{2}\sqrt{\mathrm{2}}−\mathrm{2} \\ $$$$ \\ $$$$=\mathrm{2}\left(\sqrt{\mathrm{2}}−\mathrm{1}\right) \\ $$
Commented by peter frank last updated on 25/Aug/21

$$\mathrm{good} \\ $$
Commented by rexford last updated on 26/Aug/21

$${thank}\:{you} \\ $$
