Question and Answers Forum

All Questions      Topic List

Integration Questions

Previous in All Question      Next in All Question      

Previous in Integration      Next in Integration      

Question Number 139811 by mnjuly1970 last updated on 01/May/21

𝛗:=∫_0 ^( 1) (√x) ln((1/(1βˆ’x)))dx solution: 𝛗:= ∫_0 ^( 1) (√x) Ξ£_(n=1) ^∞ (x^n /n) dx :=Ξ£_(n=1) ^∞ (1/n)∫_0 ^( 1) x^(n+(1/2)) dx := Ξ£_(n=1) ^∞ (1/(n(n+(3/2)))) = (2/3)Ξ£_(n=1) ^∞ ((1/n)βˆ’(1/(n+(3/2)))) :=(2/3){Ξ³ βˆ’Ξ³+Ξ£_(n=1) ^∞ ((1/n)βˆ’(1/(n+(3/2)))) } := (2/3) Ξ³ +(2/3) ψ(1+(3/2))=(2/3) Ξ³ +(2/3)((2/3)+ψ((3/2))) :=(2/3) Ξ³ +(4/9) +(2/3)(2+ψ((1/2))) :=(2/3) Ξ³ +((16)/9) βˆ’(2/3) Ξ³βˆ’(4/3) ln(2) :=((16)/9) βˆ’(4/3) ln(2) .....βœ“

$$ \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\boldsymbol{\phi}:=\int_{\mathrm{0}} ^{\:\mathrm{1}} \sqrt{{x}}\:{ln}\left(\frac{\mathrm{1}}{\mathrm{1}βˆ’{x}}\right){dx} \\ $$$$\:\:\:\:\:{solution}: \\ $$$$\:\:\:\:\:\:\:\boldsymbol{\phi}:=\:\int_{\mathrm{0}} ^{\:\mathrm{1}} \sqrt{{x}}\:\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{{x}^{{n}} }{{n}}\:{dx} \\ $$$$\:\:\:\:\:\:\:\:\::=\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\mathrm{1}}{{n}}\int_{\mathrm{0}} ^{\:\mathrm{1}} {x}^{{n}+\frac{\mathrm{1}}{\mathrm{2}}} {dx} \\ $$$$\:\:\:\:\:\:\:\:\::=\:\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\mathrm{1}}{{n}\left({n}+\frac{\mathrm{3}}{\mathrm{2}}\right)}\:=\:\frac{\mathrm{2}}{\mathrm{3}}\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\left(\frac{\mathrm{1}}{{n}}βˆ’\frac{\mathrm{1}}{{n}+\frac{\mathrm{3}}{\mathrm{2}}}\right) \\ $$$$\:\:\:\:\:\:\::=\frac{\mathrm{2}}{\mathrm{3}}\left\{\gamma\:βˆ’\gamma+\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\left(\frac{\mathrm{1}}{{n}}βˆ’\frac{\mathrm{1}}{{n}+\frac{\mathrm{3}}{\mathrm{2}}}\right)\:\right\} \\ $$$$\:\:\:\:\::=\:\frac{\mathrm{2}}{\mathrm{3}}\:\gamma\:+\frac{\mathrm{2}}{\mathrm{3}}\:\psi\left(\mathrm{1}+\frac{\mathrm{3}}{\mathrm{2}}\right)=\frac{\mathrm{2}}{\mathrm{3}}\:\gamma\:+\frac{\mathrm{2}}{\mathrm{3}}\left(\frac{\mathrm{2}}{\mathrm{3}}+\psi\left(\frac{\mathrm{3}}{\mathrm{2}}\right)\right) \\ $$$$\:\:\:\:\::=\frac{\mathrm{2}}{\mathrm{3}}\:\gamma\:+\frac{\mathrm{4}}{\mathrm{9}}\:+\frac{\mathrm{2}}{\mathrm{3}}\left(\mathrm{2}+\psi\left(\frac{\mathrm{1}}{\mathrm{2}}\right)\right) \\ $$$$\:\:\:\:\::=\frac{\mathrm{2}}{\mathrm{3}}\:\gamma\:+\frac{\mathrm{16}}{\mathrm{9}}\:βˆ’\frac{\mathrm{2}}{\mathrm{3}}\:\gammaβˆ’\frac{\mathrm{4}}{\mathrm{3}}\:{ln}\left(\mathrm{2}\right)\:\:\:\:\:\: \\ $$$$\:\:\:\:\:\::=\frac{\mathrm{16}}{\mathrm{9}}\:βˆ’\frac{\mathrm{4}}{\mathrm{3}}\:{ln}\left(\mathrm{2}\right)\:.....\checkmark\:\: \\ $$

Answered by mathmax by abdo last updated on 01/May/21

Ξ¦=∫_0 ^1 (√x)log((1/(1βˆ’x)))dx β‡’Ξ¦=βˆ’βˆ«_0 ^1 (√x)log(1βˆ’x)dx =_((√x)=t) βˆ’βˆ«_0 ^1 t log(1βˆ’t^2 )(2t)dt =βˆ’2∫_0 ^1 t^2 log(1βˆ’t^2 )dt =βˆ’2{ [((t^3 βˆ’1)/3)log(1βˆ’t^2 )]_0 ^1 βˆ’βˆ«_0 ^1 ((t^3 βˆ’1)/3)Γ—((βˆ’2t)/(1βˆ’t^2 ))dt} =βˆ’(4/3) ∫_0 ^1 ((t^4 βˆ’t)/(1βˆ’t^2 ))dt =(4/3)∫_0 ^1 ((t(t^3 βˆ’1))/((tβˆ’1)(t+1)))dt =(4/3) ∫_0 ^1 ((t(t^2 +t+1))/(t+1))dt =(4/3)∫_0 ^1 ((t^3 +t^2 +t)/(t+1))dt =_(t+1=y) (4/3)∫_1 ^2 (((yβˆ’1)^3 +(yβˆ’1)^2 +yβˆ’1)/y)dy =(4/3)∫_1 ^2 ((y^3 βˆ’3y^2 +3yβˆ’1+y^2 βˆ’2y+1+yβˆ’1)/y)dy =(4/3)∫_1 ^2 ((y^3 βˆ’2y^2 +2yβˆ’1)/y)dy =(4/3)∫_1 ^2 (y^2 βˆ’2y+2βˆ’(1/y))dy =(4/3)[(y^3 /3)βˆ’y^2 +2yβˆ’log∣y∣]_1 ^2 =(4/3){(8/3)βˆ’4+4βˆ’log2βˆ’(1/3)+1βˆ’2} =(4/3){(7/3)βˆ’1βˆ’log2} =(4/3)((4/3)βˆ’log2) =((16)/9)βˆ’(4/3)log2

$$\Phi=\int_{\mathrm{0}} ^{\mathrm{1}} \:\sqrt{\mathrm{x}}\mathrm{log}\left(\frac{\mathrm{1}}{\mathrm{1}βˆ’\mathrm{x}}\right)\mathrm{dx}\:\Rightarrow\Phi=βˆ’\int_{\mathrm{0}} ^{\mathrm{1}} \:\sqrt{\mathrm{x}}\mathrm{log}\left(\mathrm{1}βˆ’\mathrm{x}\right)\mathrm{dx} \\ $$$$=_{\sqrt{\mathrm{x}}=\mathrm{t}} \:\:\:βˆ’\int_{\mathrm{0}} ^{\mathrm{1}} \mathrm{t}\:\mathrm{log}\left(\mathrm{1}βˆ’\mathrm{t}^{\mathrm{2}} \right)\left(\mathrm{2t}\right)\mathrm{dt} \\ $$$$=βˆ’\mathrm{2}\int_{\mathrm{0}} ^{\mathrm{1}} \:\mathrm{t}^{\mathrm{2}} \:\mathrm{log}\left(\mathrm{1}βˆ’\mathrm{t}^{\mathrm{2}} \right)\mathrm{dt} \\ $$$$=βˆ’\mathrm{2}\left\{\:\:\left[\frac{\mathrm{t}^{\mathrm{3}} βˆ’\mathrm{1}}{\mathrm{3}}\mathrm{log}\left(\mathrm{1}βˆ’\mathrm{t}^{\mathrm{2}} \right)\right]_{\mathrm{0}} ^{\mathrm{1}} βˆ’\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{\mathrm{t}^{\mathrm{3}} βˆ’\mathrm{1}}{\mathrm{3}}Γ—\frac{βˆ’\mathrm{2t}}{\mathrm{1}βˆ’\mathrm{t}^{\mathrm{2}} }\mathrm{dt}\right\} \\ $$$$=βˆ’\frac{\mathrm{4}}{\mathrm{3}}\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\frac{\mathrm{t}^{\mathrm{4}} βˆ’\mathrm{t}}{\mathrm{1}βˆ’\mathrm{t}^{\mathrm{2}} }\mathrm{dt}\:\:=\frac{\mathrm{4}}{\mathrm{3}}\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{\mathrm{t}\left(\mathrm{t}^{\mathrm{3}} βˆ’\mathrm{1}\right)}{\left(\mathrm{t}βˆ’\mathrm{1}\right)\left(\mathrm{t}+\mathrm{1}\right)}\mathrm{dt} \\ $$$$=\frac{\mathrm{4}}{\mathrm{3}}\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{\mathrm{t}\left(\mathrm{t}^{\mathrm{2}} +\mathrm{t}+\mathrm{1}\right)}{\mathrm{t}+\mathrm{1}}\mathrm{dt}\:=\frac{\mathrm{4}}{\mathrm{3}}\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\frac{\mathrm{t}^{\mathrm{3}} \:+\mathrm{t}^{\mathrm{2}} \:+\mathrm{t}}{\mathrm{t}+\mathrm{1}}\mathrm{dt} \\ $$$$=_{\mathrm{t}+\mathrm{1}=\mathrm{y}} \:\:\:\:\frac{\mathrm{4}}{\mathrm{3}}\int_{\mathrm{1}} ^{\mathrm{2}} \:\frac{\left(\mathrm{y}βˆ’\mathrm{1}\right)^{\mathrm{3}} \:+\left(\mathrm{y}βˆ’\mathrm{1}\right)^{\mathrm{2}} \:+\mathrm{y}βˆ’\mathrm{1}}{\mathrm{y}}\mathrm{dy} \\ $$$$=\frac{\mathrm{4}}{\mathrm{3}}\int_{\mathrm{1}} ^{\mathrm{2}} \:\frac{\mathrm{y}^{\mathrm{3}} βˆ’\mathrm{3y}^{\mathrm{2}} \:+\mathrm{3y}βˆ’\mathrm{1}+\mathrm{y}^{\mathrm{2}} βˆ’\mathrm{2y}+\mathrm{1}+\mathrm{y}βˆ’\mathrm{1}}{\mathrm{y}}\mathrm{dy} \\ $$$$=\frac{\mathrm{4}}{\mathrm{3}}\int_{\mathrm{1}} ^{\mathrm{2}} \:\frac{\mathrm{y}^{\mathrm{3}} βˆ’\mathrm{2y}^{\mathrm{2}} +\mathrm{2y}βˆ’\mathrm{1}}{\mathrm{y}}\mathrm{dy} \\ $$$$=\frac{\mathrm{4}}{\mathrm{3}}\int_{\mathrm{1}} ^{\mathrm{2}} \left(\mathrm{y}^{\mathrm{2}} βˆ’\mathrm{2y}+\mathrm{2}βˆ’\frac{\mathrm{1}}{\mathrm{y}}\right)\mathrm{dy} \\ $$$$=\frac{\mathrm{4}}{\mathrm{3}}\left[\frac{\mathrm{y}^{\mathrm{3}} }{\mathrm{3}}βˆ’\mathrm{y}^{\mathrm{2}} \:+\mathrm{2y}βˆ’\mathrm{log}\mid\mathrm{y}\mid\right]_{\mathrm{1}} ^{\mathrm{2}} \\ $$$$=\frac{\mathrm{4}}{\mathrm{3}}\left\{\frac{\mathrm{8}}{\mathrm{3}}βˆ’\mathrm{4}+\mathrm{4}βˆ’\mathrm{log2}βˆ’\frac{\mathrm{1}}{\mathrm{3}}+\mathrm{1}βˆ’\mathrm{2}\right\} \\ $$$$=\frac{\mathrm{4}}{\mathrm{3}}\left\{\frac{\mathrm{7}}{\mathrm{3}}βˆ’\mathrm{1}βˆ’\mathrm{log2}\right\}\:=\frac{\mathrm{4}}{\mathrm{3}}\left(\frac{\mathrm{4}}{\mathrm{3}}βˆ’\mathrm{log2}\right)\:=\frac{\mathrm{16}}{\mathrm{9}}βˆ’\frac{\mathrm{4}}{\mathrm{3}}\mathrm{log2} \\ $$

Commented by mnjuly1970 last updated on 01/May/21

thx sir max ...

$${thx}\:{sir}\:{max}\:... \\ $$

Commented by mathmax by abdo last updated on 02/May/21

you are welcome sir

$$\mathrm{you}\:\mathrm{are}\:\mathrm{welcome}\:\mathrm{sir} \\ $$

Answered by Ar Brandon last updated on 16/May/21

Ο†=∫_0 ^1 (√x)ln((1/(1βˆ’x)))dx=βˆ’βˆ«_0 ^1 (√x)ln(1βˆ’x)dx ∫_0 ^1 x^(mβˆ’1) (1βˆ’x)^(nβˆ’1) dx=Ξ²(m,n)=((Ξ“(m)Ξ“(n))/(Ξ“(m+n))) lnΞ²(m,n)=lnΞ“(m)+lnΞ“(n)βˆ’lnΞ“(m+n) (1/(Ξ²(m,n)))βˆ™((βˆ‚Ξ²(m,n))/βˆ‚n)=ψ(n)βˆ’Οˆ(m+n) β‡’((βˆ‚Ξ²((3/2),1))/βˆ‚n)=Ξ²((3/2),1){ψ(1)βˆ’Οˆ((5/2))} =((Ξ“((3/2)))/(Ξ“((5/2)))){βˆ’Ξ³βˆ’((2/3)+2βˆ’Ξ³βˆ’2ln2)} Ο†=βˆ’(2/3)(2ln2βˆ’(8/3))=((16)/9)βˆ’((4ln2)/3)

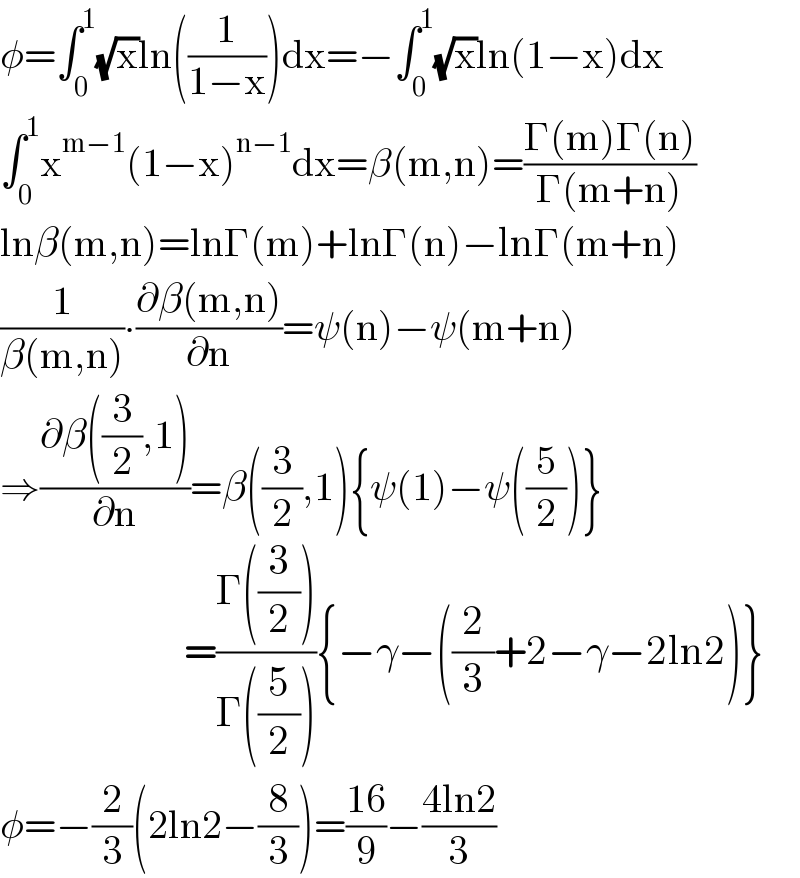
$$\phi=\int_{\mathrm{0}} ^{\mathrm{1}} \sqrt{\mathrm{x}}\mathrm{ln}\left(\frac{\mathrm{1}}{\mathrm{1}βˆ’\mathrm{x}}\right)\mathrm{dx}=βˆ’\int_{\mathrm{0}} ^{\mathrm{1}} \sqrt{\mathrm{x}}\mathrm{ln}\left(\mathrm{1}βˆ’\mathrm{x}\right)\mathrm{dx} \\ $$$$\int_{\mathrm{0}} ^{\mathrm{1}} \mathrm{x}^{\mathrm{m}βˆ’\mathrm{1}} \left(\mathrm{1}βˆ’\mathrm{x}\right)^{\mathrm{n}βˆ’\mathrm{1}} \mathrm{dx}=\beta\left(\mathrm{m},\mathrm{n}\right)=\frac{\Gamma\left(\mathrm{m}\right)\Gamma\left(\mathrm{n}\right)}{\Gamma\left(\mathrm{m}+\mathrm{n}\right)} \\ $$$$\mathrm{ln}\beta\left(\mathrm{m},\mathrm{n}\right)=\mathrm{ln}\Gamma\left(\mathrm{m}\right)+\mathrm{ln}\Gamma\left(\mathrm{n}\right)βˆ’\mathrm{ln}\Gamma\left(\mathrm{m}+\mathrm{n}\right) \\ $$$$\frac{\mathrm{1}}{\beta\left(\mathrm{m},\mathrm{n}\right)}\centerdot\frac{\partial\beta\left(\mathrm{m},\mathrm{n}\right)}{\partial\mathrm{n}}=\psi\left(\mathrm{n}\right)βˆ’\psi\left(\mathrm{m}+\mathrm{n}\right) \\ $$$$\Rightarrow\frac{\partial\beta\left(\frac{\mathrm{3}}{\mathrm{2}},\mathrm{1}\right)}{\partial\mathrm{n}}=\beta\left(\frac{\mathrm{3}}{\mathrm{2}},\mathrm{1}\right)\left\{\psi\left(\mathrm{1}\right)βˆ’\psi\left(\frac{\mathrm{5}}{\mathrm{2}}\right)\right\} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\frac{\Gamma\left(\frac{\mathrm{3}}{\mathrm{2}}\right)}{\Gamma\left(\frac{\mathrm{5}}{\mathrm{2}}\right)}\left\{βˆ’\gammaβˆ’\left(\frac{\mathrm{2}}{\mathrm{3}}+\mathrm{2}βˆ’\gammaβˆ’\mathrm{2ln2}\right)\right\} \\ $$$$\phi=βˆ’\frac{\mathrm{2}}{\mathrm{3}}\left(\mathrm{2ln2}βˆ’\frac{\mathrm{8}}{\mathrm{3}}\right)=\frac{\mathrm{16}}{\mathrm{9}}βˆ’\frac{\mathrm{4ln2}}{\mathrm{3}} \\ $$

Commented by mnjuly1970 last updated on 01/May/21

grateful mr brandon...

$$\:\:{grateful}\:{mr}\:{brandon}... \\ $$

Commented by SLVR last updated on 01/May/21

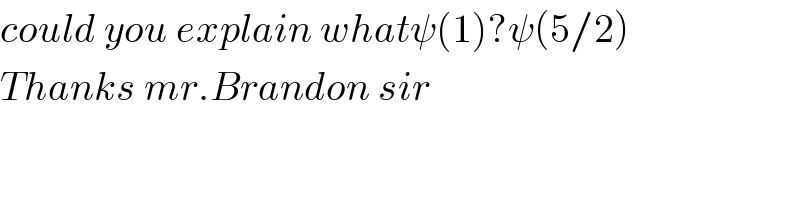
could you explain whatψ(1)?ψ(5/2) Thanks mr.Brandon sir

$${could}\:{you}\:{explain}\:{what}\psi\left(\mathrm{1}\right)?\psi\left(\mathrm{5}/\mathrm{2}\right) \\ $$$${Thanks}\:{mr}.{Brandon}\:{sir} \\ $$

Commented by Ar Brandon last updated on 01/May/21

Youβ€²re welcome Sir

$$\mathrm{You}'\mathrm{re}\:\mathrm{welcome}\:\mathrm{Sir} \\ $$

Commented by Ar Brandon last updated on 01/May/21

Ξ“β€²(x)=Ξ“(x)ψ(x)β‡’((Ξ“β€²(x))/(Ξ“(x)))=ψ(x) ψ(s+1)=(1/s)+ψ(s) , ψ((1/2))=βˆ’Ξ³βˆ’2ln2 ψ(x)=βˆ’Ξ³+Ξ£_(n=0) ^∞ ((1/(n+1))βˆ’(1/(n+x))) β‡’Οˆ(1)=βˆ’Ξ³ ψ(x) represents digamma function.

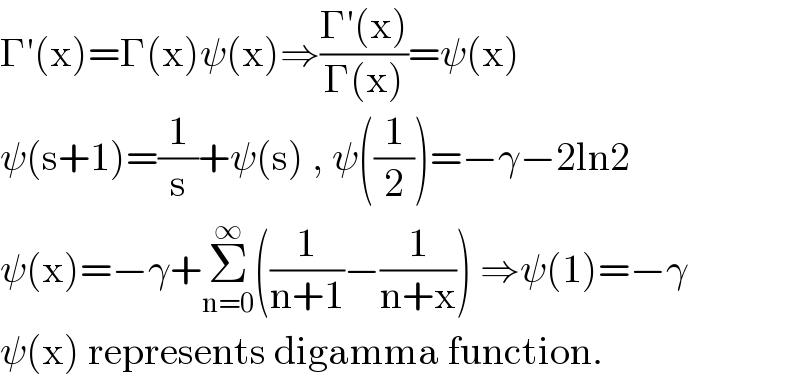
$$\Gamma'\left(\mathrm{x}\right)=\Gamma\left(\mathrm{x}\right)\psi\left(\mathrm{x}\right)\Rightarrow\frac{\Gamma'\left(\mathrm{x}\right)}{\Gamma\left(\mathrm{x}\right)}=\psi\left(\mathrm{x}\right) \\ $$$$\psi\left(\mathrm{s}+\mathrm{1}\right)=\frac{\mathrm{1}}{\mathrm{s}}+\psi\left(\mathrm{s}\right)\:,\:\psi\left(\frac{\mathrm{1}}{\mathrm{2}}\right)=βˆ’\gammaβˆ’\mathrm{2ln2} \\ $$$$\psi\left(\mathrm{x}\right)=βˆ’\gamma+\underset{\mathrm{n}=\mathrm{0}} {\overset{\infty} {\sum}}\left(\frac{\mathrm{1}}{\mathrm{n}+\mathrm{1}}βˆ’\frac{\mathrm{1}}{\mathrm{n}+\mathrm{x}}\right)\:\Rightarrow\psi\left(\mathrm{1}\right)=βˆ’\gamma \\ $$$$\psi\left(\mathrm{x}\right)\:\mathrm{represents}\:\mathrm{digamma}\:\mathrm{function}. \\ $$

Commented by SLVR last updated on 01/May/21

So....kind you..sir Great...explanation God...bless you for ever

$${So}....{kind}\:{you}..{sir}\:\:{Great}...{explanation} \\ $$$${God}...{bless}\:{you}\:{for}\:{ever} \\ $$

Commented by Ar Brandon last updated on 01/May/21

Thanks!

$$\mathrm{Thanks}! \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com