
Question Number 217290 by mnjuly1970 last updated on 08/Mar/25
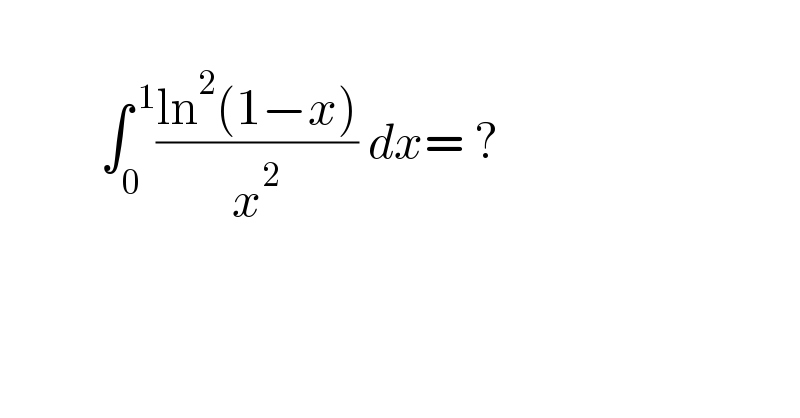
$$ \\ $$$$\:\:\:\:\:\:\:\:\:\:\int_{\mathrm{0}} ^{\:\mathrm{1}} \frac{\mathrm{ln}^{\mathrm{2}} \left(\mathrm{1}−{x}\right)}{{x}^{\mathrm{2}} }\:{dx}=\:? \\ $$$$ \\ $$$$ \\ $$
Answered by profcedricjunior last updated on 09/Mar/25
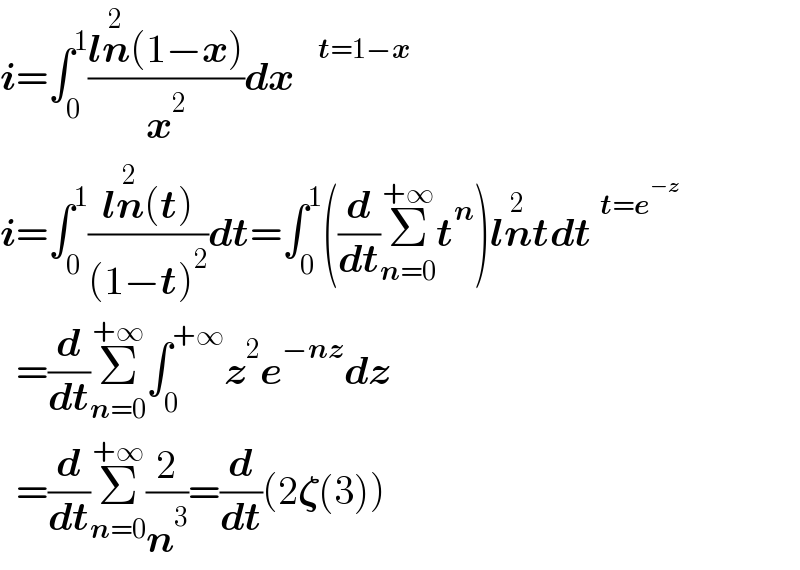
$$\boldsymbol{{i}}=\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\boldsymbol{{l}}\overset{\mathrm{2}} {\boldsymbol{{n}}}\left(\mathrm{1}−\boldsymbol{{x}}\right)}{\boldsymbol{{x}}^{\mathrm{2}} }\boldsymbol{{dx}}\:\:\:\overset{\boldsymbol{{t}}=\mathrm{1}−\boldsymbol{{x}}} {\:} \\ $$$$\boldsymbol{{i}}=\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\boldsymbol{{l}}\overset{\mathrm{2}} {\boldsymbol{{n}}}\left(\boldsymbol{{t}}\right)}{\left(\mathrm{1}−\boldsymbol{{t}}\right)^{\mathrm{2}} }\boldsymbol{{dt}}=\int_{\mathrm{0}} ^{\mathrm{1}} \left(\frac{\boldsymbol{{d}}}{\boldsymbol{{dt}}}\underset{\boldsymbol{{n}}=\mathrm{0}} {\overset{+\infty} {\sum}}\boldsymbol{{t}}^{\boldsymbol{{n}}} \right)\boldsymbol{{l}}\overset{\mathrm{2}} {\boldsymbol{{n}tdt}}\:\overset{\boldsymbol{{t}}=\boldsymbol{{e}}^{−\boldsymbol{{z}}} } {\:} \\ $$$$\:\:=\frac{\boldsymbol{{d}}}{\boldsymbol{{dt}}}\underset{\boldsymbol{{n}}=\mathrm{0}} {\overset{+\infty} {\sum}}\int_{\mathrm{0}} ^{+\infty} \boldsymbol{{z}}^{\mathrm{2}} \boldsymbol{{e}}^{−\boldsymbol{{nz}}} \boldsymbol{{dz}} \\ $$$$\:\:=\frac{\boldsymbol{{d}}}{\boldsymbol{{dt}}}\underset{\boldsymbol{{n}}=\mathrm{0}} {\overset{+\infty} {\sum}}\frac{\mathrm{2}}{\boldsymbol{{n}}^{\mathrm{3}} }=\frac{\boldsymbol{{d}}}{\boldsymbol{{dt}}}\left(\mathrm{2}\boldsymbol{\zeta}\left(\mathrm{3}\right)\right) \\ $$
Commented by Ghisom last updated on 10/Mar/25

$$\mathrm{this}\:\mathrm{is}\:\mathrm{nonsense} \\ $$$$\frac{{d}}{{dt}}\left(\mathrm{2}\zeta\left(\mathrm{3}\right)\right)=\mathrm{0} \\ $$
Answered by vnm last updated on 09/Mar/25

$$\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\mathrm{ln}^{\mathrm{2}} \left(\mathrm{1}−{x}\right)}{{x}^{\mathrm{2}} }{dx}=\int_{\mathrm{0}} ^{\mathrm{1}} \mathrm{ln}^{\mathrm{2}} \left(\mathrm{1}−{x}\right)\frac{{d}}{{dx}}\left(−\frac{\mathrm{1}}{{x}}\right){dx}= \\ $$$$−\frac{\mathrm{1}}{{x}}\mathrm{ln}^{\mathrm{2}} \left(\mathrm{1}−{x}\right)\mid_{\mathrm{0}} ^{\mathrm{1}} +\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\mathrm{1}}{{x}}\frac{{d}}{{dx}}\mathrm{ln}^{\mathrm{2}} \left(\mathrm{1}−{x}\right)\:{dx}= \\ $$$$−\frac{\mathrm{1}}{{x}}\mathrm{ln}^{\mathrm{2}} \left(\mathrm{1}−{x}\right)\mid_{\mathrm{0}} ^{\mathrm{1}} −\mathrm{2}\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\mathrm{ln}\left(\mathrm{1}−{x}\right)}{{x}\left(\mathrm{1}−{x}\right)}{dx}= \\ $$$$−\frac{\mathrm{1}}{{x}}\mathrm{ln}^{\mathrm{2}} \left(\mathrm{1}−{x}\right)\mid_{\mathrm{0}} ^{\mathrm{1}} −\mathrm{2}\int_{\mathrm{0}} ^{\mathrm{1}} \mathrm{ln}\left(\mathrm{1}−{x}\right)\left(\frac{\mathrm{1}}{\mathrm{1}−{x}}+\frac{\mathrm{1}}{{x}}\right){dx}= \\ $$$$−\frac{\mathrm{1}}{{x}}\mathrm{ln}^{\mathrm{2}} \left(\mathrm{1}−{x}\right)\mid_{\mathrm{0}} ^{\mathrm{1}} +\mathrm{ln}^{\mathrm{2}} \left(\mathrm{1}−{x}\right)\mid_{\mathrm{0}} ^{\mathrm{1}} −\mathrm{2}\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\mathrm{ln}\left(\mathrm{1}−{x}\right)}{{x}}{dx}= \\ $$$$\left(\mathrm{1}−\frac{\mathrm{1}}{{x}}\right)\mathrm{ln}^{\mathrm{2}} \left(\mathrm{1}−{x}\right)\mid_{\mathrm{0}} ^{\mathrm{1}} −\mathrm{2}\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\mathrm{ln}\left(\mathrm{1}−{x}\right)}{{x}}{dx}={I} \\ $$$$\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\left(\mathrm{1}−\frac{\mathrm{1}}{{x}}\right)\mathrm{ln}^{\mathrm{2}} \left(\mathrm{1}−{x}\right)=\underset{{x}\rightarrow\mathrm{1}} {\mathrm{lim}}\left(\mathrm{1}−\frac{\mathrm{1}}{{x}}\right)\mathrm{ln}^{\mathrm{2}} \left(\mathrm{1}−{x}\right)=\mathrm{0} \\ $$$$−\mathrm{2}\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\mathrm{ln}\left(\mathrm{1}−{x}\right)}{{x}}{dx}=−\mathrm{2}\int_{\mathrm{0}} ^{\mathrm{1}} \frac{−\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{{x}^{{n}} }{{n}}}{{x}}{dx}= \\ $$$$\mathrm{2}\int_{\mathrm{0}} ^{\mathrm{1}} \underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{{x}^{{n}−\mathrm{1}} }{{n}}{dx}=\mathrm{2}\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{x}^{{n}−\mathrm{1}} }{{n}}{dx}=\mathrm{2}\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\left(\frac{{x}^{{n}} }{{n}^{\mathrm{2}} }\mid_{\mathrm{0}} ^{\mathrm{1}} \right)=\mathrm{2}\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\mathrm{1}}{{n}^{\mathrm{2}} }=\mathrm{2}\frac{\pi^{\mathrm{2}} }{\mathrm{6}}=\frac{\pi^{\mathrm{2}} }{\mathrm{3}} \\ $$$${I}=\frac{\pi^{\mathrm{2}} }{\mathrm{3}} \\ $$$$ \\ $$
Commented by mnjuly1970 last updated on 10/Mar/25

$${thx}\:{alot} \\ $$
Commented by Tawa11 last updated on 10/Mar/25

$$\mathrm{Weldone}\:\mathrm{sir}. \\ $$
Answered by mr W last updated on 12/Mar/25
![with t=1−x ∫_0 ^1 ((ln^2 (1−x))/x^2 )dx =∫_0 ^1 ((ln^2 t)/((1−t)^2 ))dt =∫_0 ^1 ln^2 t (Σ_(n=1) ^∞ nt^(n−1) )dt =Σ_(n=1) ^∞ n∫_0 ^1 (ln^2 t) t^(n−1) dt =Σ_(n=0) ^∞ (n+1)∫_0 ^1 (ln^2 t) t^n dt I=∫_0 ^1 (ln^2 t) t^n dt =(1/(n+1))∫_0 ^1 (ln^2 t)dt^(n+1) =(1/(n+1)){[ln^2 t t^(n+1) ]_0 ^1 −2∫_0 ^1 ln t t^n dt} =−(2/(n+1))∫_0 ^1 ln t t^n dt =−(2/((n+1)^2 ))∫_0 ^1 ln t dt^(n+1) =−(2/((n+1)^2 )){[ln t t^(n+1) ]_0 ^1 −∫_0 ^1 t^n dt} =(2/((n+1)^2 ))∫_0 ^1 t^n dt =(2/((n+1)^3 ))[t^(n+1) ]_0 ^1 =(2/((n+1)^3 )) ∫_0 ^1 ((ln^2 (1−x))/x^2 )dx =Σ_(n=0) ^∞ ((2(n+1))/((n+1)^3 )) =2Σ_(n=0) ^∞ (1/((n+1)^2 )) =2Σ_(n=1) ^∞ (1/n^2 )=2ζ(2)=2×(π^2 /6)=(π^2 /3) ✓](Q217376.png)
$${with}\:{t}=\mathrm{1}−{x} \\ $$$$\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\mathrm{ln}^{\mathrm{2}} \:\left(\mathrm{1}−{x}\right)}{{x}^{\mathrm{2}} }{dx} \\ $$$$=\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\mathrm{ln}^{\mathrm{2}} \:{t}}{\left(\mathrm{1}−{t}\right)^{\mathrm{2}} }{dt} \\ $$$$=\int_{\mathrm{0}} ^{\mathrm{1}} \mathrm{ln}^{\mathrm{2}} \:{t}\:\left(\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}{nt}^{{n}−\mathrm{1}} \right){dt} \\ $$$$=\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}{n}\int_{\mathrm{0}} ^{\mathrm{1}} \left(\mathrm{ln}^{\mathrm{2}} \:{t}\right)\:{t}^{{n}−\mathrm{1}} {dt} \\ $$$$=\underset{{n}=\mathrm{0}} {\overset{\infty} {\sum}}\left({n}+\mathrm{1}\right)\int_{\mathrm{0}} ^{\mathrm{1}} \left(\mathrm{ln}^{\mathrm{2}} \:{t}\right)\:{t}^{{n}} {dt} \\ $$$$ \\ $$$${I}=\int_{\mathrm{0}} ^{\mathrm{1}} \left(\mathrm{ln}^{\mathrm{2}} \:{t}\right)\:{t}^{{n}} {dt} \\ $$$$\:=\frac{\mathrm{1}}{{n}+\mathrm{1}}\int_{\mathrm{0}} ^{\mathrm{1}} \left(\mathrm{ln}^{\mathrm{2}} \:{t}\right){dt}^{{n}+\mathrm{1}} \\ $$$$\:=\frac{\mathrm{1}}{{n}+\mathrm{1}}\left\{\left[\mathrm{ln}^{\mathrm{2}} \:{t}\:{t}^{{n}+\mathrm{1}} \right]_{\mathrm{0}} ^{\mathrm{1}} −\mathrm{2}\int_{\mathrm{0}} ^{\mathrm{1}} \mathrm{ln}\:{t}\:{t}^{{n}} \:{dt}\right\} \\ $$$$\:=−\frac{\mathrm{2}}{{n}+\mathrm{1}}\int_{\mathrm{0}} ^{\mathrm{1}} \mathrm{ln}\:{t}\:{t}^{{n}} \:{dt} \\ $$$$\:=−\frac{\mathrm{2}}{\left({n}+\mathrm{1}\right)^{\mathrm{2}} }\int_{\mathrm{0}} ^{\mathrm{1}} \mathrm{ln}\:{t}\:{dt}^{{n}+\mathrm{1}} \\ $$$$\:=−\frac{\mathrm{2}}{\left({n}+\mathrm{1}\right)^{\mathrm{2}} }\left\{\left[\mathrm{ln}\:{t}\:{t}^{{n}+\mathrm{1}} \right]_{\mathrm{0}} ^{\mathrm{1}} −\int_{\mathrm{0}} ^{\mathrm{1}} {t}^{{n}} \:{dt}\right\} \\ $$$$\:=\frac{\mathrm{2}}{\left({n}+\mathrm{1}\right)^{\mathrm{2}} }\int_{\mathrm{0}} ^{\mathrm{1}} {t}^{{n}} \:{dt} \\ $$$$\:=\frac{\mathrm{2}}{\left({n}+\mathrm{1}\right)^{\mathrm{3}} }\left[{t}^{{n}+\mathrm{1}} \right]_{\mathrm{0}} ^{\mathrm{1}} \\ $$$$\:=\frac{\mathrm{2}}{\left({n}+\mathrm{1}\right)^{\mathrm{3}} } \\ $$$$ \\ $$$$\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\mathrm{ln}^{\mathrm{2}} \:\left(\mathrm{1}−{x}\right)}{{x}^{\mathrm{2}} }{dx} \\ $$$$=\underset{{n}=\mathrm{0}} {\overset{\infty} {\sum}}\frac{\mathrm{2}\left({n}+\mathrm{1}\right)}{\left({n}+\mathrm{1}\right)^{\mathrm{3}} } \\ $$$$=\mathrm{2}\underset{{n}=\mathrm{0}} {\overset{\infty} {\sum}}\frac{\mathrm{1}}{\left({n}+\mathrm{1}\right)^{\mathrm{2}} } \\ $$$$=\mathrm{2}\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\mathrm{1}}{{n}^{\mathrm{2}} }=\mathrm{2}\zeta\left(\mathrm{2}\right)=\mathrm{2}×\frac{\pi^{\mathrm{2}} }{\mathrm{6}}=\frac{\pi^{\mathrm{2}} }{\mathrm{3}}\:\:\checkmark \\ $$
Commented by Tawa11 last updated on 14/Mar/25

$$\mathrm{Weldone}\:\mathrm{sir}. \\ $$
Answered by MrGaster last updated on 14/Mar/25
![t=1−x∧x∈[0,1],t∈[0,1]∧dx−dt⇒^(∫_0 ^( 1) ((ln^2 (1−x))/x^2 ) dx) ∫_0 ^1 ((ln^2 t)/((1−t)^2 ))dt ∫_0 ^1 ((ln^2 t)/((1−t)^2 ))dt=∫_0 ^1 ln^2 tΣ_(n=0) ^∞ (n+1)t^n dt (1/((1−t)^2 ))=Σ_(n=0) ^∞ (n+1)t^n ,∣t∣<1⇒Σ_(n=0) ^∞ (n+1)∫_0 ^1 t^n ln^2 t dt ∫_0 ^1 t^n ln^2 t dt=(2/((n+1)^3 )) ∴2Σ_(n=0) ^∞ ((n+1)/((n+1)^3 ))=2Σ_(n=0) ^∞ (1/((n+1)^2 ))=(1/k^2 )2∙(π^2 /6)=(π^2 /3)](Q217451.png)
$${t}=\mathrm{1}−{x}\wedge{x}\in\left[\mathrm{0},\mathrm{1}\right],{t}\in\left[\mathrm{0},\mathrm{1}\right]\wedge{dx}−{dt}\overset{\int_{\mathrm{0}} ^{\:\mathrm{1}} \frac{\mathrm{ln}^{\mathrm{2}} \left(\mathrm{1}−{x}\right)}{{x}^{\mathrm{2}} }\:{dx}} {\Rightarrow}\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\mathrm{ln}^{\mathrm{2}} {t}}{\left(\mathrm{1}−{t}\right)^{\mathrm{2}} }{dt} \\ $$$$\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\mathrm{ln}^{\mathrm{2}} {t}}{\left(\mathrm{1}−{t}\right)^{\mathrm{2}} }{dt}=\int_{\mathrm{0}} ^{\mathrm{1}} \mathrm{ln}^{\mathrm{2}} {t}\underset{{n}=\mathrm{0}} {\overset{\infty} {\sum}}\left({n}+\mathrm{1}\right){t}^{{n}} {dt} \\ $$$$\frac{\mathrm{1}}{\left(\mathrm{1}−{t}\right)^{\mathrm{2}} }=\underset{{n}=\mathrm{0}} {\overset{\infty} {\sum}}\left({n}+\mathrm{1}\right){t}^{{n}} ,\mid{t}\mid<\mathrm{1}\Rightarrow\underset{{n}=\mathrm{0}} {\overset{\infty} {\sum}}\left({n}+\mathrm{1}\right)\int_{\mathrm{0}} ^{\mathrm{1}} {t}^{{n}} \mathrm{ln}^{\mathrm{2}} {t}\:{dt} \\ $$$$\int_{\mathrm{0}} ^{\mathrm{1}} {t}^{{n}} \mathrm{ln}^{\mathrm{2}} {t}\:{dt}=\frac{\mathrm{2}}{\left({n}+\mathrm{1}\right)^{\mathrm{3}} } \\ $$$$\therefore\mathrm{2}\underset{{n}=\mathrm{0}} {\overset{\infty} {\sum}}\frac{{n}+\mathrm{1}}{\left({n}+\mathrm{1}\right)^{\mathrm{3}} }=\mathrm{2}\underset{{n}=\mathrm{0}} {\overset{\infty} {\sum}}\frac{\mathrm{1}}{\left({n}+\mathrm{1}\right)^{\mathrm{2}} }=\frac{\mathrm{1}}{{k}^{\mathrm{2}} }\mathrm{2}\centerdot\frac{\pi^{\mathrm{2}} }{\mathrm{6}}=\frac{\pi^{\mathrm{2}} }{\mathrm{3}} \\ $$
