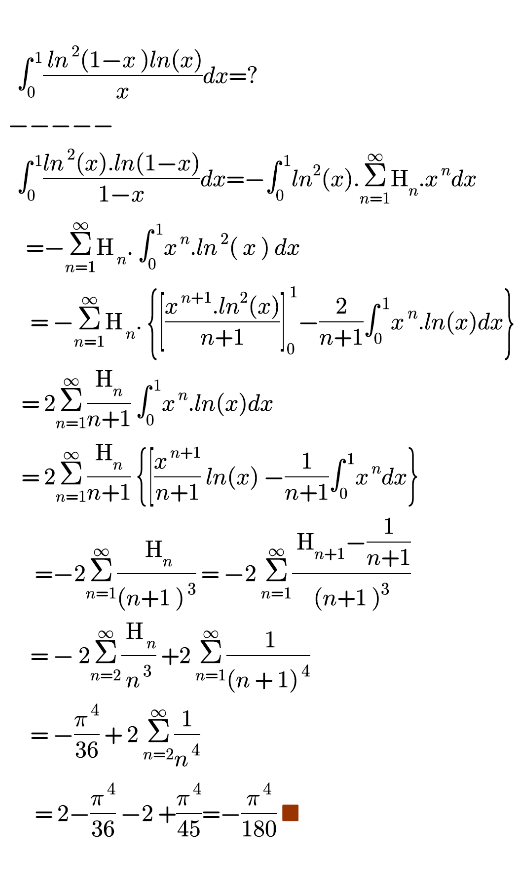Question Number 160252 by mnjuly1970 last updated on 26/Nov/21
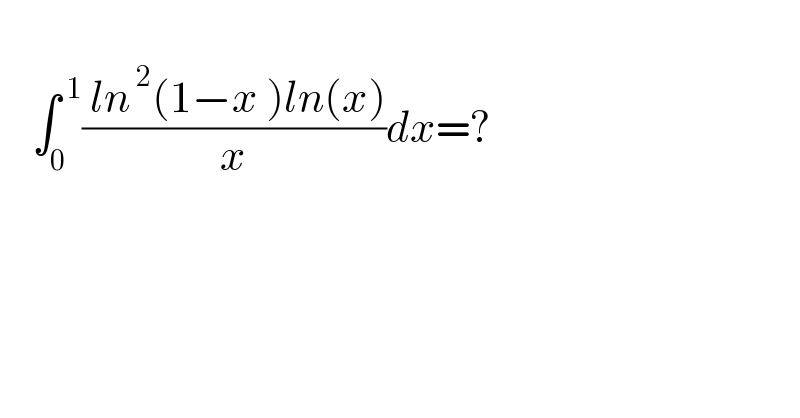
$$ \\ $$$$\:\:\:\:\int_{\mathrm{0}} ^{\:\mathrm{1}} \frac{\:{ln}^{\:\mathrm{2}} \left(\mathrm{1}−{x}\:\right){ln}\left({x}\right)}{{x}}{dx}=? \\ $$$$ \\ $$
Answered by TheSupreme last updated on 26/Nov/21
![I= ∫_0 ^1 ln^2 (1−x)((ln(x))/x)dx=(1/2)ln^2 (1−x)ln^2 (x)−∫_0 ^1 −((ln(1−x))/(1−x))ln^2 (x)dx= t=1−x →x=1−t→dx=−dt =(1/2)ln^2 (1−x)ln^2 (x)−∫_0 ^1 ((ln(t))/t)ln^2 (1−t)dt =(1/2)ln^2 (1−x)ln^2 (x)−∫_0 ^1 ((ln(t)ln^2 (1−t))/t)dt 2I=(1/2)ln^2 (1−x)ln^2 (x)]_0 ^1 ∫_0 ^1 ln^2 (1−x)((ln(x))/x)=(1/4)ln^2 (1−x) ln^2 (x)]_0 ^1 lim_(x→0) (1/4)ln^2 (1−x)ln^2 (x)=(1/4)x^2 ln^2 (x)=0 I=0](Q160258.png)
$${I}= \\ $$$$\int_{\mathrm{0}} ^{\mathrm{1}} {ln}^{\mathrm{2}} \left(\mathrm{1}−{x}\right)\frac{{ln}\left({x}\right)}{{x}}{dx}=\frac{\mathrm{1}}{\mathrm{2}}{ln}^{\mathrm{2}} \left(\mathrm{1}−{x}\right){ln}^{\mathrm{2}} \left({x}\right)−\int_{\mathrm{0}} ^{\mathrm{1}} −\frac{{ln}\left(\mathrm{1}−{x}\right)}{\mathrm{1}−{x}}{ln}^{\mathrm{2}} \left({x}\right){dx}= \\ $$$${t}=\mathrm{1}−{x}\:\rightarrow{x}=\mathrm{1}−{t}\rightarrow{dx}=−{dt} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}{ln}^{\mathrm{2}} \left(\mathrm{1}−{x}\right){ln}^{\mathrm{2}} \left({x}\right)−\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{ln}\left({t}\right)}{{t}}{ln}^{\mathrm{2}} \left(\mathrm{1}−{t}\right){dt} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}{ln}^{\mathrm{2}} \left(\mathrm{1}−{x}\right){ln}^{\mathrm{2}} \left({x}\right)−\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{ln}\left({t}\right){ln}^{\mathrm{2}} \left(\mathrm{1}−{t}\right)}{{t}}{dt} \\ $$$$\left.\mathrm{2}{I}=\frac{\mathrm{1}}{\mathrm{2}}{ln}^{\mathrm{2}} \left(\mathrm{1}−{x}\right){ln}^{\mathrm{2}} \left({x}\right)\right]_{\mathrm{0}} ^{\mathrm{1}} \\ $$$$\left.\int_{\mathrm{0}} ^{\mathrm{1}} {ln}^{\mathrm{2}} \left(\mathrm{1}−{x}\right)\frac{{ln}\left({x}\right)}{{x}}=\frac{\mathrm{1}}{\mathrm{4}}{ln}^{\mathrm{2}} \left(\mathrm{1}−{x}\right)\:{ln}^{\mathrm{2}} \left({x}\right)\right]_{\mathrm{0}} ^{\mathrm{1}} \\ $$$${li}\underset{{x}\rightarrow\mathrm{0}} {{m}}\:\frac{\mathrm{1}}{\mathrm{4}}{ln}^{\mathrm{2}} \left(\mathrm{1}−{x}\right){ln}^{\mathrm{2}} \left({x}\right)=\frac{\mathrm{1}}{\mathrm{4}}{x}^{\mathrm{2}} {ln}^{\mathrm{2}} \left({x}\right)=\mathrm{0} \\ $$$${I}=\mathrm{0} \\ $$
Commented by mr W last updated on 27/Nov/21
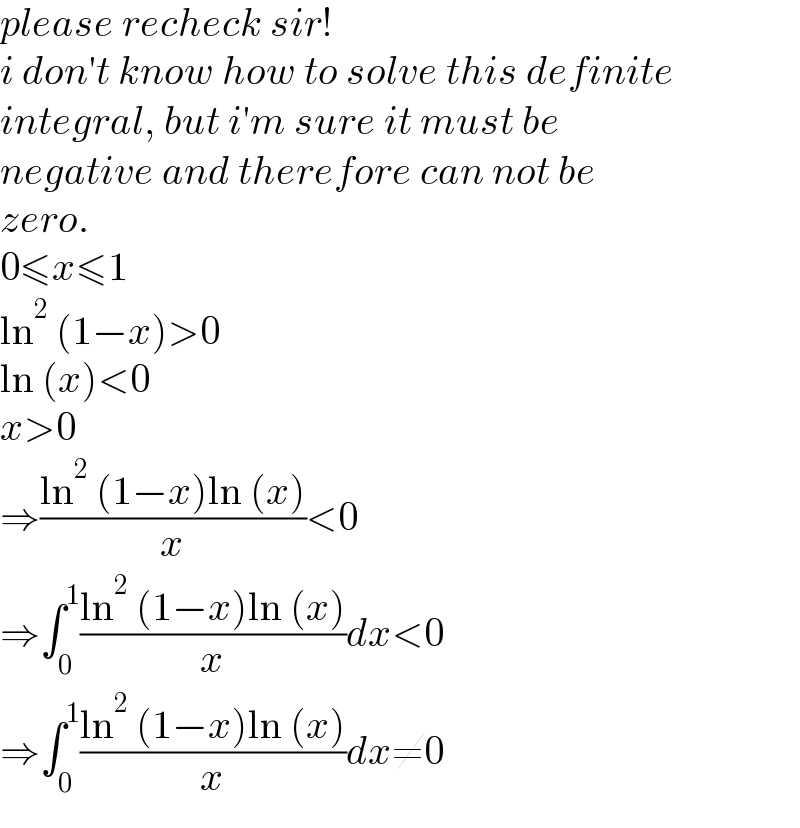
$${please}\:{recheck}\:{sir}! \\ $$$${i}\:{don}'{t}\:{know}\:{how}\:{to}\:{solve}\:{this}\:{definite} \\ $$$${integral},\:{but}\:{i}'{m}\:{sure}\:{it}\:{must}\:{be} \\ $$$${negative}\:{and}\:{therefore}\:{can}\:{not}\:{be}\: \\ $$$${zero}. \\ $$$$\mathrm{0}\leqslant{x}\leqslant\mathrm{1} \\ $$$$\mathrm{ln}^{\mathrm{2}} \:\left(\mathrm{1}−{x}\right)>\mathrm{0} \\ $$$$\mathrm{ln}\:\left({x}\right)<\mathrm{0} \\ $$$${x}>\mathrm{0} \\ $$$$\Rightarrow\frac{\mathrm{ln}^{\mathrm{2}} \:\left(\mathrm{1}−{x}\right)\mathrm{ln}\:\left({x}\right)}{{x}}<\mathrm{0} \\ $$$$\Rightarrow\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\mathrm{ln}^{\mathrm{2}} \:\left(\mathrm{1}−{x}\right)\mathrm{ln}\:\left({x}\right)}{{x}}{dx}<\mathrm{0} \\ $$$$\Rightarrow\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\mathrm{ln}^{\mathrm{2}} \:\left(\mathrm{1}−{x}\right)\mathrm{ln}\:\left({x}\right)}{{x}}{dx}\neq\mathrm{0} \\ $$
Commented by MJS_new last updated on 27/Nov/21
![∫_0 ^1 ((ln^2 (1−x) ln x)/x)dx= [by parts] =[(1/2)ln^2 x ln^2 (1−x)]_0 ^1 +∫_0 ^1 ((ln^2 x ln (1−x))/(1−x))dx= [t=1−x → dx=−dt] =[(1/2)ln^2 x ln^2 (1−x)]_0 ^1 −∫_1 ^0 ((ln^2 (1−t) ln t)/t)dt= =[(1/2)ln^2 x ln^2 (1−x)]_0 ^1 +∫_0 ^1 ((ln^2 (1−t) ln t)/t)dt so we have I=[(1/2)ln^2 x ln^2 (1−x)]_0 ^1 +I we cannot solve it this way (or am I too tired to understand anything? it′s 2:30 a.m.)](Q160266.png)
$$\underset{\mathrm{0}} {\overset{\mathrm{1}} {\int}}\:\frac{\mathrm{ln}^{\mathrm{2}} \:\left(\mathrm{1}−{x}\right)\:\mathrm{ln}\:{x}}{{x}}{dx}= \\ $$$$\:\:\:\:\:\left[\mathrm{by}\:\mathrm{parts}\right] \\ $$$$=\left[\frac{\mathrm{1}}{\mathrm{2}}\mathrm{ln}^{\mathrm{2}} \:{x}\:\mathrm{ln}^{\mathrm{2}} \:\left(\mathrm{1}−{x}\right)\right]_{\mathrm{0}} ^{\mathrm{1}} +\underset{\mathrm{0}} {\overset{\mathrm{1}} {\int}}\:\frac{\mathrm{ln}^{\mathrm{2}} \:{x}\:\mathrm{ln}\:\left(\mathrm{1}−{x}\right)}{\mathrm{1}−{x}}{dx}= \\ $$$$\:\:\:\:\:\left[{t}=\mathrm{1}−{x}\:\rightarrow\:{dx}=−{dt}\right] \\ $$$$=\left[\frac{\mathrm{1}}{\mathrm{2}}\mathrm{ln}^{\mathrm{2}} \:{x}\:\mathrm{ln}^{\mathrm{2}} \:\left(\mathrm{1}−{x}\right)\right]_{\mathrm{0}} ^{\mathrm{1}} −\underset{\mathrm{1}} {\overset{\mathrm{0}} {\int}}\:\frac{\mathrm{ln}^{\mathrm{2}} \:\left(\mathrm{1}−{t}\right)\:\mathrm{ln}\:{t}}{{t}}{dt}= \\ $$$$=\left[\frac{\mathrm{1}}{\mathrm{2}}\mathrm{ln}^{\mathrm{2}} \:{x}\:\mathrm{ln}^{\mathrm{2}} \:\left(\mathrm{1}−{x}\right)\right]_{\mathrm{0}} ^{\mathrm{1}} +\underset{\mathrm{0}} {\overset{\mathrm{1}} {\int}}\:\frac{\mathrm{ln}^{\mathrm{2}} \:\left(\mathrm{1}−{t}\right)\:\mathrm{ln}\:{t}}{{t}}{dt} \\ $$$$\mathrm{so}\:\mathrm{we}\:\mathrm{have} \\ $$$${I}=\left[\frac{\mathrm{1}}{\mathrm{2}}\mathrm{ln}^{\mathrm{2}} \:{x}\:\mathrm{ln}^{\mathrm{2}} \:\left(\mathrm{1}−{x}\right)\right]_{\mathrm{0}} ^{\mathrm{1}} +{I} \\ $$$$\mathrm{we}\:\mathrm{cannot}\:\mathrm{solve}\:\mathrm{it}\:\mathrm{this}\:\mathrm{way}\:\left(\mathrm{or}\:\mathrm{am}\:\mathrm{I}\:\mathrm{too}\:\mathrm{tired}\right. \\ $$$$\left.\mathrm{to}\:\mathrm{understand}\:\mathrm{anything}?\:\mathrm{it}'\mathrm{s}\:\mathrm{2}:\mathrm{30}\:\mathrm{a}.\mathrm{m}.\right) \\ $$
Commented by MJS_new last updated on 27/Nov/21
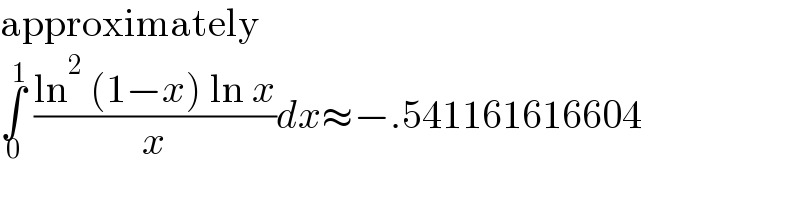
$$\mathrm{approximately} \\ $$$$\underset{\mathrm{0}} {\overset{\mathrm{1}} {\int}}\:\frac{\mathrm{ln}^{\mathrm{2}} \:\left(\mathrm{1}−{x}\right)\:\mathrm{ln}\:{x}}{{x}}{dx}\approx−.\mathrm{541161616604} \\ $$
Answered by mnjuly1970 last updated on 27/Nov/21