
Question Number 206962 by Ghisom last updated on 01/May/24
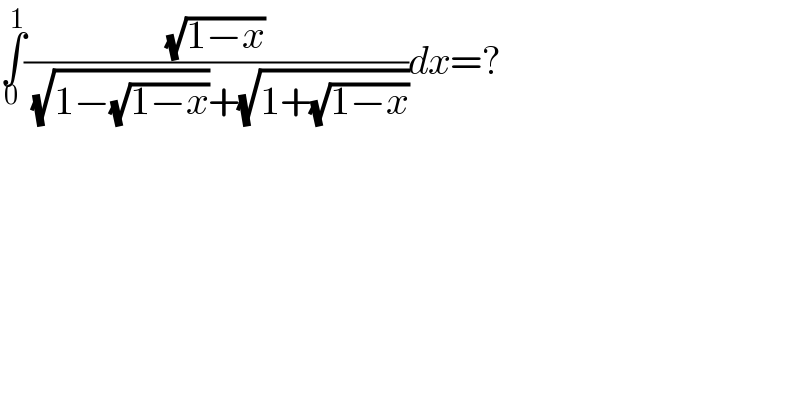
$$\underset{\mathrm{0}} {\overset{\mathrm{1}} {\int}}\frac{\sqrt{\mathrm{1}−{x}}}{\:\sqrt{\mathrm{1}−\sqrt{\mathrm{1}−{x}}}+\sqrt{\mathrm{1}+\sqrt{\mathrm{1}−{x}}}}{dx}=? \\ $$
Answered by lepuissantcedricjunior last updated on 02/May/24
![∫_0 ^1 ((√(1−x))/( (√(1−(√(1−x))))+(√(1+(√(1−x))))))dx=k k=∫_0 ^1 ((√(1−x))/(((√(1−(√(1−x)))))^2 −((√(1+(√(1−x)))))^2 ))×(((√(1−(√(1−x))))−(√(1+(√(1−x)))))/1) dx k=∫_0 ^1 (((√(1−x))((√(1−(√(1−x))))−(√(1+(√(1−x))))))/(−2(√(1−(√x)))))dx k=−(1/2)∫_0 ^1 ((√(1−(√(1−x)))))dx+(1/2)∫_0 ^1 ((√(1+(√(1−x)))))dx posons { ((1−(√(1−x))=u^2 )),((1+(√(1−x))=v^2 )) :}=> { ((x=1−(1−u^2 )^2 )),((x=1−(v^2 −1)^2 )) :} => { ((dx=2u(1−u^2 )du)),((dx=−2v(v^2 −1)dv)) :} qd: { ( { ((x→0)),((x→1)) :}),( { ((x→1)),((x→0)) :}) :}=> { ( { ((u→0)),((u→1)) :}),( { ((v→1)),((v→2)) :}) :} k=−∫_0 ^1 u^2 (1−u^2 )du−∫_1 ^2 v^2 (v^2 −1)dv =[(1/3)u^3 −(1/5)u^5 ]_0 ^1 −[(1/5)v^5 −(1/3)v^3 ]_1 ^2 =[(1/3)−(1/5)−((32)/5)+(8/3)+(1/5)−(1/3)] =(8/3)−((32)/5)=((40−96)/(15))=−((56)/(15))](Q206985.png)
$$\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\sqrt{\mathrm{1}−\boldsymbol{{x}}}}{\:\sqrt{\mathrm{1}−\sqrt{\mathrm{1}−\boldsymbol{{x}}}}+\sqrt{\mathrm{1}+\sqrt{\mathrm{1}−\boldsymbol{{x}}}}}\boldsymbol{{dx}}=\boldsymbol{{k}} \\ $$$$\boldsymbol{{k}}=\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\sqrt{\mathrm{1}−\boldsymbol{{x}}}}{\left(\sqrt{\mathrm{1}−\sqrt{\mathrm{1}−\boldsymbol{{x}}}}\right)^{\mathrm{2}} −\left(\sqrt{\mathrm{1}+\sqrt{\mathrm{1}−\boldsymbol{{x}}}}\right)^{\mathrm{2}} }×\frac{\sqrt{\mathrm{1}−\sqrt{\mathrm{1}−\boldsymbol{{x}}}}−\sqrt{\mathrm{1}+\sqrt{\mathrm{1}−\boldsymbol{{x}}}}}{\mathrm{1}}\:\boldsymbol{{dx}} \\ $$$$\boldsymbol{{k}}=\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\sqrt{\mathrm{1}−\boldsymbol{{x}}}\left(\sqrt{\mathrm{1}−\sqrt{\mathrm{1}−\boldsymbol{{x}}}}−\sqrt{\mathrm{1}+\sqrt{\mathrm{1}−{x}}}\right)}{−\mathrm{2}\sqrt{\mathrm{1}−\sqrt{\boldsymbol{{x}}}}}\boldsymbol{{dx}} \\ $$$$\boldsymbol{{k}}=−\frac{\mathrm{1}}{\mathrm{2}}\int_{\mathrm{0}} ^{\mathrm{1}} \left(\sqrt{\mathrm{1}−\sqrt{\mathrm{1}−\boldsymbol{{x}}}}\right)\boldsymbol{{dx}}+\frac{\mathrm{1}}{\mathrm{2}}\int_{\mathrm{0}} ^{\mathrm{1}} \left(\sqrt{\mathrm{1}+\sqrt{\mathrm{1}−\boldsymbol{{x}}}}\right)\boldsymbol{{dx}} \\ $$$$\boldsymbol{{posons}}\:\begin{cases}{\mathrm{1}−\sqrt{\mathrm{1}−\boldsymbol{{x}}}=\boldsymbol{{u}}^{\mathrm{2}} }\\{\mathrm{1}+\sqrt{\mathrm{1}−\boldsymbol{{x}}}=\boldsymbol{{v}}^{\mathrm{2}} }\end{cases}=>\begin{cases}{\boldsymbol{{x}}=\mathrm{1}−\left(\mathrm{1}−\boldsymbol{{u}}^{\mathrm{2}} \right)^{\mathrm{2}} }\\{\boldsymbol{{x}}=\mathrm{1}−\left(\boldsymbol{{v}}^{\mathrm{2}} −\mathrm{1}\right)^{\mathrm{2}} }\end{cases}\:=>\begin{cases}{\boldsymbol{{dx}}=\mathrm{2}\boldsymbol{{u}}\left(\mathrm{1}−\boldsymbol{{u}}^{\mathrm{2}} \right)\boldsymbol{{du}}}\\{\boldsymbol{{dx}}=−\mathrm{2}\boldsymbol{{v}}\left(\boldsymbol{{v}}^{\mathrm{2}} −\mathrm{1}\right)\boldsymbol{{dv}}}\end{cases} \\ $$$$\boldsymbol{{qd}}:\begin{cases}{\begin{cases}{\boldsymbol{{x}}\rightarrow\mathrm{0}}\\{\boldsymbol{{x}}\rightarrow\mathrm{1}}\end{cases}}\\{\begin{cases}{\boldsymbol{{x}}\rightarrow\mathrm{1}}\\{\boldsymbol{{x}}\rightarrow\mathrm{0}}\end{cases}}\end{cases}=>\begin{cases}{\begin{cases}{\boldsymbol{{u}}\rightarrow\mathrm{0}}\\{\boldsymbol{{u}}\rightarrow\mathrm{1}}\end{cases}}\\{\begin{cases}{\boldsymbol{{v}}\rightarrow\mathrm{1}}\\{\boldsymbol{{v}}\rightarrow\mathrm{2}}\end{cases}}\end{cases} \\ $$$$\boldsymbol{{k}}=−\int_{\mathrm{0}} ^{\mathrm{1}} \boldsymbol{{u}}^{\mathrm{2}} \left(\mathrm{1}−\boldsymbol{{u}}^{\mathrm{2}} \right)\boldsymbol{{du}}−\int_{\mathrm{1}} ^{\mathrm{2}} \boldsymbol{{v}}^{\mathrm{2}} \left(\boldsymbol{{v}}^{\mathrm{2}} −\mathrm{1}\right)\boldsymbol{{dv}} \\ $$$$\:\:\:=\left[\frac{\mathrm{1}}{\mathrm{3}}\boldsymbol{{u}}^{\mathrm{3}} −\frac{\mathrm{1}}{\mathrm{5}}\boldsymbol{{u}}^{\mathrm{5}} \right]_{\mathrm{0}} ^{\mathrm{1}} −\left[\frac{\mathrm{1}}{\mathrm{5}}\boldsymbol{{v}}^{\mathrm{5}} −\frac{\mathrm{1}}{\mathrm{3}}\boldsymbol{{v}}^{\mathrm{3}} \right]_{\mathrm{1}} ^{\mathrm{2}} \\ $$$$\:\:\:=\left[\frac{\mathrm{1}}{\mathrm{3}}−\frac{\mathrm{1}}{\mathrm{5}}−\frac{\mathrm{32}}{\mathrm{5}}+\frac{\mathrm{8}}{\mathrm{3}}+\frac{\mathrm{1}}{\mathrm{5}}−\frac{\mathrm{1}}{\mathrm{3}}\right] \\ $$$$\:\:\:=\frac{\mathrm{8}}{\mathrm{3}}−\frac{\mathrm{32}}{\mathrm{5}}=\frac{\mathrm{40}−\mathrm{96}}{\mathrm{15}}=−\frac{\mathrm{56}}{\mathrm{15}} \\ $$
Commented by Frix last updated on 02/May/24
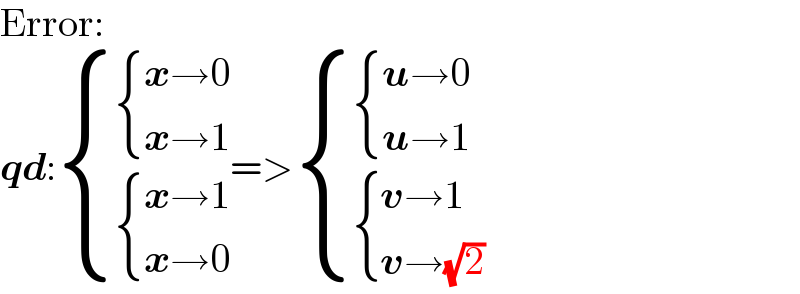
$$\mathrm{Error}: \\ $$$$\boldsymbol{{qd}}:\begin{cases}{\begin{cases}{\boldsymbol{{x}}\rightarrow\mathrm{0}}\\{\boldsymbol{{x}}\rightarrow\mathrm{1}}\end{cases}}\\{\begin{cases}{\boldsymbol{{x}}\rightarrow\mathrm{1}}\\{\boldsymbol{{x}}\rightarrow\mathrm{0}}\end{cases}}\end{cases}=>\begin{cases}{\begin{cases}{\boldsymbol{{u}}\rightarrow\mathrm{0}}\\{\boldsymbol{{u}}\rightarrow\mathrm{1}}\end{cases}}\\{\begin{cases}{\boldsymbol{{v}}\rightarrow\mathrm{1}}\\{\boldsymbol{{v}}\rightarrow\sqrt{\mathrm{2}}}\end{cases}}\end{cases} \\ $$
Answered by Frix last updated on 02/May/24
![∫_0 ^1 ((√(1−x))/( (√(1−(√(1−x))))+(√(1+(√(1−x))))))×(((√(1+(√(1−x))))−(√(1−(√(1−x)))))/( (√(1+(√(1−x))))−(√(1−(√(1−x))))))dx= =∫_0 ^1 (((√(1+(√(1−x))))−(√(1−(√(1−x)))))/2)dx= =(1/2)∫_0 ^1 (√(1+(√(1−x))))dx−(1/2)∫_0 ^1 (√(1−(√(1−x))))dx (1/2)∫_0 ^1 (√(1+(√(1−x))))dx= t=(√(1+(√(1−x)))) ⇔ x=2t^2 −t^4 ∧t≥1 ⇒ dx=4(t−t^3 )dt =2∫_( (√2)) ^1 t^2 −t^4 dt=2[(t^3 /3)−(t^5 /5)]_(√2) ^1 =((4(1+(√2)))/(15)) (1/2)∫_0 ^1 (√(1−(√(1−x))))dx= t=(√(1−(√(1−x)))) ⇔ x=2t^2 −t^4 ∧0≤t≤1 ⇒ dx=4(t−t^3 )dt =2∫_0 ^1 t^2 −t^4 dt=2[(t^3 /3)−(t^5 /5)]_0 ^1 =(4/(15)) ⇒ ∫_0 ^1 ((√(1−x))/( (√(1−(√(1−x))))+(√(1+(√(1−x))))))dx=((4(√2))/(15))](Q206967.png)
$$\underset{\mathrm{0}} {\overset{\mathrm{1}} {\int}}\frac{\sqrt{\mathrm{1}−{x}}}{\:\sqrt{\mathrm{1}−\sqrt{\mathrm{1}−{x}}}+\sqrt{\mathrm{1}+\sqrt{\mathrm{1}−{x}}}}×\frac{\sqrt{\mathrm{1}+\sqrt{\mathrm{1}−{x}}}−\sqrt{\mathrm{1}−\sqrt{\mathrm{1}−{x}}}}{\:\sqrt{\mathrm{1}+\sqrt{\mathrm{1}−{x}}}−\sqrt{\mathrm{1}−\sqrt{\mathrm{1}−{x}}}}{dx}= \\ $$$$=\underset{\mathrm{0}} {\overset{\mathrm{1}} {\int}}\frac{\sqrt{\mathrm{1}+\sqrt{\mathrm{1}−{x}}}−\sqrt{\mathrm{1}−\sqrt{\mathrm{1}−{x}}}}{\mathrm{2}}{dx}= \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\underset{\mathrm{0}} {\overset{\mathrm{1}} {\int}}\sqrt{\mathrm{1}+\sqrt{\mathrm{1}−{x}}}{dx}−\frac{\mathrm{1}}{\mathrm{2}}\underset{\mathrm{0}} {\overset{\mathrm{1}} {\int}}\sqrt{\mathrm{1}−\sqrt{\mathrm{1}−{x}}}{dx} \\ $$$$ \\ $$$$\frac{\mathrm{1}}{\mathrm{2}}\underset{\mathrm{0}} {\overset{\mathrm{1}} {\int}}\sqrt{\mathrm{1}+\sqrt{\mathrm{1}−{x}}}{dx}= \\ $$$$\:\:\:\:\:{t}=\sqrt{\mathrm{1}+\sqrt{\mathrm{1}−{x}}}\:\Leftrightarrow\:{x}=\mathrm{2}{t}^{\mathrm{2}} −{t}^{\mathrm{4}} \wedge{t}\geqslant\mathrm{1} \\ $$$$\:\:\:\:\:\Rightarrow\:{dx}=\mathrm{4}\left({t}−{t}^{\mathrm{3}} \right){dt} \\ $$$$=\mathrm{2}\underset{\:\sqrt{\mathrm{2}}} {\overset{\mathrm{1}} {\int}}{t}^{\mathrm{2}} −{t}^{\mathrm{4}} {dt}=\mathrm{2}\left[\frac{{t}^{\mathrm{3}} }{\mathrm{3}}−\frac{{t}^{\mathrm{5}} }{\mathrm{5}}\right]_{\sqrt{\mathrm{2}}} ^{\mathrm{1}} =\frac{\mathrm{4}\left(\mathrm{1}+\sqrt{\mathrm{2}}\right)}{\mathrm{15}} \\ $$$$ \\ $$$$\frac{\mathrm{1}}{\mathrm{2}}\underset{\mathrm{0}} {\overset{\mathrm{1}} {\int}}\sqrt{\mathrm{1}−\sqrt{\mathrm{1}−{x}}}{dx}= \\ $$$$\:\:\:\:\:{t}=\sqrt{\mathrm{1}−\sqrt{\mathrm{1}−{x}}}\:\Leftrightarrow\:{x}=\mathrm{2}{t}^{\mathrm{2}} −{t}^{\mathrm{4}} \wedge\mathrm{0}\leqslant{t}\leqslant\mathrm{1} \\ $$$$\:\:\:\:\:\Rightarrow\:{dx}=\mathrm{4}\left({t}−{t}^{\mathrm{3}} \right){dt} \\ $$$$=\mathrm{2}\underset{\mathrm{0}} {\overset{\mathrm{1}} {\int}}{t}^{\mathrm{2}} −{t}^{\mathrm{4}} {dt}=\mathrm{2}\left[\frac{{t}^{\mathrm{3}} }{\mathrm{3}}−\frac{{t}^{\mathrm{5}} }{\mathrm{5}}\right]_{\mathrm{0}} ^{\mathrm{1}} =\frac{\mathrm{4}}{\mathrm{15}} \\ $$$$ \\ $$$$\Rightarrow \\ $$$$\underset{\mathrm{0}} {\overset{\mathrm{1}} {\int}}\frac{\sqrt{\mathrm{1}−{x}}}{\:\sqrt{\mathrm{1}−\sqrt{\mathrm{1}−{x}}}+\sqrt{\mathrm{1}+\sqrt{\mathrm{1}−{x}}}}{dx}=\frac{\mathrm{4}\sqrt{\mathrm{2}}}{\mathrm{15}} \\ $$
Commented by Ghisom last updated on 02/May/24

$$\mathrm{thank}\:\mathrm{you} \\ $$
